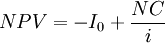Net Present Value (NPV)
(→Discount rate) |
|||
| (672 intermediate revisions by 2 users not shown) | |||
| Line 2: | Line 2: | ||
== Abstract == | == Abstract == | ||
| − | |||
| − | + | The financial appraisal is a method used to evaluate whether a proposed project, program, or portfolio is worthwhile by considering the benefits and costs that result from its execution <ref name="Häcker" />. Investment decisions of the management are critical to a company since it decides the future of the company <ref name="LOCK"/>. This article discusses the ''' net present value (NPV)''' method which is widely used in financial appraisal. NPV is a dynamic financial appraisal method that considers the time value of money by applying discounting to all future payments during the investment period <ref name="Häcker" />. In simple terms, NPV is the difference between the project's future incoming and outgoing cash flows at present time. NPV calculation begins with determining the net cash flows from the difference of future incoming and outgoing cash flows for each period of an investment. Once the net cash flow is calculated for each period, the present value (PV) is obtained by discounting using a suitable discount rate. NPV is the cumulative of all the present values of the future net cash flows <ref name="Häcker" /> <ref name="Ferrari" />. | |
| − | == Big | + | Firstly, this article discusses the idea behind the NPV method. Then this introduces the NPV calculation method and describes the importance of choosing the suitable discount rate. Then it highlights the decision criteria behind NPV and explains it with real-life applications. Also, it discusses the other NPV formulas for special cases such as annuity and perpetuity. Finally, it critically reflects on the limitations of this method and briefly highlights the key references used in this article. |
| − | + | ||
| + | == Big idea == | ||
| + | |||
| + | According to ISO 21502, a business case is a documented justification to support decision-making about the commitment to a project, program or portfolio <ref name="ISO_21502"/>. Development of a business case is a critical point in a project, program or portfolio where it uses to obtain the approval by presenting the benefits, cost, and risk associated with alternative options <ref name="PRINCE2"/> <ref name="PRINCE2_program"/> <ref name="PRINCE2_portfolio"/>. The business case is produced by the client or the sponsor of the project, program or portfolio and the directorate of an organization uses this document to accept or reject the case. <ref name="Ing" />. In a business case, financial appraisal plays a key role to answer the main questions of whether an investment should be made and which project should be chosen among a selection of different alternatives. Because the task of financial appraisal is to predict the financial effects of planned investment and to present the data to get critical investment decisions <ref name="Häcker" />. NPV method is one of the most frequently used approaches in the financial appraisal of a project, program, or portfolio <ref name="Häcker" /> <ref name="Konstantin" />. | ||
=== What is NPV? === | === What is NPV? === | ||
| − | + | ||
| + | NPV is the present value of the future net cash flows of an investment. <ref name="Häcker" />. In other words, NPV is the difference between the present value of all future incoming and outgoing cash flows. The NPV of a project is calculated based on different assumptions such as <ref name="Žižlavský" /> <ref name="Bora" /> <ref name="Poggensee" /> : | ||
| + | |||
| + | * Money in the future will not be worth the same amount of money today. Therefore, all the future cash flows are converted to present value by using a suitable discount rate. | ||
| + | * The discount rate is constant throughout the lifetime of the project. | ||
| + | * The cash obtained by a project is reinvested immediately by the discount rate. | ||
| + | * All the incoming and outgoing cash flows except the initial investment occur at the end of each period. | ||
| + | |||
| + | NPV is a widely used method in the financial appraisal that has the following benefits <ref name="Bora" /> : | ||
| + | |||
| + | * NPV considers the concept of the time value of money by obtaining the present value of future net cash flows. | ||
| + | * NPV calculation considers the complete life cycle of an investment using all the future cash flows. | ||
| + | * It has the additive property. That means the possibility to sum the NPV of individual projects to evaluate the project portfolio. | ||
| + | * For businesses, the NPV method facilitates the decision-making process by identifying whether a project, program, or portfolio is profit-making or loss-making. | ||
=== Time value of money === | === Time value of money === | ||
| − | |||
| − | === | + | NPV is one of the most precise financial appraisal methods since it considers the time value of money in the analysis. Time value of money means that a sum of money is worth more now than the same sum of money in the future <ref name="LOCK" /> <ref name="Žižlavský" /> <ref name="Bora" /> . That is because the money you have now can use to make more money by buying something now and selling it later for more money, running a business, or depositing the money in the bank and earning interest. Since money can grow only through investing, investment delay is an opportunity cost. That means not having the money right now also includes the loss of additional income. Future money is also less valuable because inflation erodes its buying power. Moreover, receiving money in the future rather than now may involve some risk and uncertainty regarding its recovery. For these reasons, future cash flows are worth less than the present cash flows. NPV assesses the profitability of a given project on the basis that cash flow in the future is not worth the same as a cash flow today. The time value of money is recognized in NPV by applying discounting to all future cash flows generated during the investment period using a suitable discount rate<ref name="Konstantin" />. |
| − | + | ||
| − | + | === Discount rate === | |
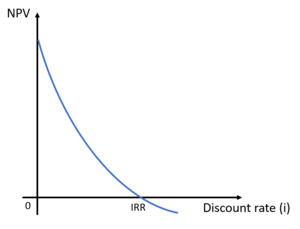
| − | + | [[File:NPV-vs-i.png|thumb|300px|right|Figure 1: NPV vs. Discount rate (i). Own creation inspired from <ref name="Ferrari" />.]] | |
| − | + | ||
| − | + | ||
| − | + | ||
| − | < | + | |
| − | + | ||
| − | + | ||
| + | The discount rate is the rate used to determine the present value of future cash flows in NPV calculation. The choice of the discount rate can dramatically change the NPV since the NPV is an inverse function of the discount rate (i) <ref name="Ferrari" />. The selection of discount rate will be company-specific since it is related to how the company finds its funds <ref name="Žižlavský" />. If an investment is financed completely with equity, the discount rate can be obtained from a cost of equity. On the other hand, if the investment is completely debt-financed it can be analyzed using the cost of debt. If an investment depends on both equity and debt financing, the discount rate is the weighted average cost of capital (WACC) and can be calculated as the weighted average of the cost of equity and cost of debt <ref name="Žižlavský" />. WACC is often used in companies as the discount rate <ref name="Häcker" />. For small projects, where it is hard to identify the equity and debt used for financing a single project, the WACC of the whole company is usually assumed as the discount rate <ref name="Žižlavský" />. | ||
| − | + | However, it is appropriate to use higher discount rates to reflect other factors such as risk, the opportunity cost of money invested in the infrastructure and etc <ref name="Ferrari" />. Also, NPV calculated using variable discount rates may correctly interpret the situation than one calculated from a constant discount rate. <ref name="Häcker" />. | |
| + | === How to calculate NPV? === | ||
| + | The following formulas use these common variables <ref name="Häcker" />: | ||
| − | + | {| class="wikitable" | |
| + | |+ style="caption-side:bottom; color:black;"|''Table 1: List of variables use in NPV formulas. Own creation inspired from <ref name="Häcker" />.'' | ||
| + | |- | ||
| + | ! style="color:black" | Variable | ||
| + | ! style="color:black" | Meaning | ||
| + | |- | ||
| + | |<math display="inline">P V</math> | ||
| + | |Present value | ||
| + | |- | ||
| + | |<math>t</math> | ||
| + | |Time of the cashflow | ||
| + | |- | ||
| + | |<math>C I _{t}</math> | ||
| + | |Cash inflows in period t | ||
| + | |- | ||
| + | |<math>C O _{t}</math> | ||
| + | |Cash outflows in period t | ||
| + | |- | ||
| + | |<math>{\left(C I _{t}-C O _{t}\right)} = N C _{t}</math> | ||
| + | |Net cash flow in period t | ||
| + | |- | ||
| + | |<math>N C</math> | ||
| + | |Constant net cash flow for all periods | ||
| + | |- | ||
| + | |<math>I_{o}</math> | ||
| + | |Investment in t=0 | ||
| + | |- | ||
| + | |<math>L_{n}</math> | ||
| + | |Liquidation proceeds in t=n | ||
| + | |- | ||
| + | |<math>i</math> | ||
| + | |The discount rate | ||
| + | |- | ||
| + | |<math>n</math> | ||
| + | |Total number of periods | ||
| + | |} | ||
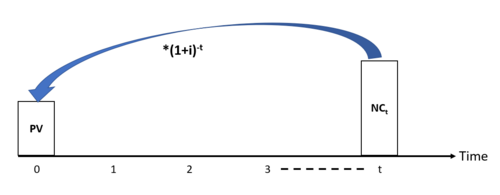
| + | [[File:PV_New.png|thumb|500px|right|Figure 2: Projection of a future net cash flow in time t into the present value. Own creation inspired from <ref name="Poggensee" />.]] | ||
| + | In order to consider the time value of money, the NPV method considers the value of an investment at the present time by projecting all future cash flows into a key figure at the time today. This projection of the value that a future cash flow has in the present is called '''present value (PV)'''. To get the present value of a future net cash flow in period t, it is discounted using a discount rate (i) as follows: | ||
| + | <math>P V=\frac{\left(C I _{t}-C O _{t}\right)}{(1+i)^{t}}</math> <ref name="Häcker" /> | ||
| − | + | '''Example 1:''' | |
| − | + | If a project is expected to receive a net cash flow of $5,000 with 10% discount rate after 4 years, its present value can be calculated as: | |
| − | + | <math>P V=\frac{\left(C I _{t}-C O _{t}\right)}{(1+i)^{t}}</math> <ref name="Häcker" /> | |
| − | + | <math>P V=\frac{5000}{(1+0.1)^{4}} = $3415 </math> | |
| − | |||
| + | NPV combines these projections of all net cash flows to assess the overall value of a project from the present view. Therefore the NPV is given by: | ||
| − | + | <math>N P V=\sum_{t=0}^{n} \frac{\left(C I _{t}-C O _{t}\right)}{(1+i)^{t}}</math> <ref name="Häcker" /> | |
| − | + | ||
| − | + | ||
| − | + | [[File:NPV_New1.png|thumb|500px|right|Figure 3: Illustration of the calculation procedure of NPV. Own creation inspired from <ref name="Poggensee" />]] | |
| + | The difference between the cash inflows and cash outflows, <math>{\left(C I _{t}-C O _{t}\right)}</math> gives the net cash flow, <math>N C _{t}</math> of the corresponding period. For investments which are characterized by initial investment, <math>I_{0}</math>, subtracting it from the present value of the net cash flows gives the net present value. If a possible final payment, <math>L_{n}</math>, for the liquidation of the investment in t = n is also considered, the NPV can be calculated as follows <ref name="Häcker" />: | ||
| − | <math>N P V=-I_{0}+\sum_{t=1}^{n} \frac{N C | + | <math>N P V=-I_{0}+\sum_{t=1}^{n} \frac{N C _{t}}{(1+i)^{t}}+\frac{L_{n}}{(1+i)^{n}}</math> <ref name="Häcker" /> |
| + | As a summary, the following steps are recommended for the calculation of the net present value <ref name="Häcker" />: | ||
| − | + | # Determination of the initial investment, <math>I_{0}</math> . | |
| + | # Estimate the expected net cash flows, <math>N C _{t}</math> for each period. | ||
| + | # Selection of the constant discount rate, <math>i</math>. | ||
| + | # Determination of the present values by discounting the net cash flows and finally obtaining the summation. | ||
| + | # Subtraction of the initial investment, <math>I_{0}</math> from the summation of the present values. This gives the net present value. | ||
| − | + | '''Example 2:''' | |
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | A company decides to invest $100,000 for a glass manufacturing plant which is expected to give the following cash flows. Assume the discount rate is 10%. Calculate the NPV of the project. | |
| − | + | {| class="wikitable" | |
| − | + | |+ style="caption-side:bottom; color:black;"|''Table 2: Expected net cash flows of the glass manufacturing plant. Own creation.'' | |
| − | + | |- | |
| + | ! style="color:black" | | ||
| + | ! style="color:black" | Now | ||
| + | ! style="color:black" | Year 1 | ||
| + | ! style="color:black" | Year 2 | ||
| + | ! style="color:black" | Year 3 | ||
| + | |- | ||
| + | |Initial Investment | ||
| + | |$100,000 | ||
| + | | | ||
| + | | | ||
| + | | | ||
| + | |- | ||
| + | |Running costs | ||
| + | | | ||
| + | |$60,000 | ||
| + | |$90,000 | ||
| + | |$90,000 | ||
| + | |- | ||
| + | |Revenues | ||
| + | | | ||
| + | |$80,000 | ||
| + | |$100,000 | ||
| + | |$120,000 | ||
| + | |- | ||
| + | |Liquidation proceeds | ||
| + | | | ||
| + | | | ||
| + | | | ||
| + | |$140,000 | ||
| + | |} | ||
| − | + | NPV can be calculated using the formula as follows. | |
| − | == | + | <math>N P V=-I_{0}+\sum_{t=1}^{n} \frac{N C _{t}}{(1+i)^{t}}+\frac{L_{n}}{(1+i)^{n}}</math> <ref name="Häcker" /> |
| − | + | ||
| − | + | ||
| − | === | + | <math>N P V=-100,000+ \frac{(-60,000+80,000)}{(1+0.1)^{1}}+ \frac{(-90,000+100,000)}{(1+0.1)^{2}}+\frac{(-90,000+120,000)}{(1+0.1)^{3}}+\frac{140,000}{(1+0.1)^{3}}</math> |
| + | |||
| + | <math>N P V=-100,000+ 18182+ 8265+ 22539+ 105184 = $54170</math> | ||
=== Decision rule === | === Decision rule === | ||
| − | This | + | |
| + | NPV is used to assess the attractiveness of the investment and it shows how much value an investment or project adds to the firm and the decision to accept or reject the investment is taken based on the following decision rule <ref name="Häcker" />. | ||
| + | |||
| + | {| class="wikitable" | ||
| + | |+ style="caption-side:bottom; color:black;"|''Table 3: Decision criteria of NPV. Own creation inspired from <ref name="Häcker" />.'' | ||
| + | |- | ||
| + | ! style="color:black" | Condition | ||
| + | ! style="color:black" | Idea | ||
| + | ! style="color:black" | Decision | ||
| + | |- | ||
| + | |If NPV > 0 | ||
| + | |Investment increases the value (profit) of the company in the amount of the NPV. | ||
| + | |Investment can be accepted. | ||
| + | |- | ||
| + | |If NPV = 0 | ||
| + | |No increase or decrease in value of the company. | ||
| + | |This project adds no monetary value. Difficult to get the decision whether to accept or reject the project. Decisions should be based on other criteria. | ||
| + | |- | ||
| + | |If NPV < 0 | ||
| + | |Investment would decrease value (profit) in an amount equal to the negative NPV. | ||
| + | |The investment can be rejected. | ||
| + | |} | ||
| + | |||
| + | As a summary, the following two profitability criteria must be met for the acceptance of an investment <ref name="Häcker" />: | ||
| + | |||
| + | * '''Absolute profitability''': NPV of an investment option must be positive or at least zero (NPV≥ 0). | ||
| + | |||
| + | * '''Relative profitability''': If there are several positive NPV options, the one with the highest NPV should be selected. | ||
| + | |||
| + | == Other NPV formulas for special scenarios == | ||
| + | |||
| + | Note that this section also uses the same variables as listed in Table 1 above. | ||
| + | |||
| + | === NPV of an annuity === | ||
| + | |||
| + | Net cash flows can be interpreted as an annuity, if they are constant, equidistant and occur at the end of each period <ref name="Häcker" />. Using the formula for the present value of an ordinary annuity, NPV can be calculated as follows <ref name="Häcker" />: | ||
| + | |||
| + | <math>N P V=-I_{0}+N C \cdot\left[\frac{(1+i)^{n}-1}{i \cdot(1+i)^{n}}\right]+\frac{L_{n}}{(1+i)^{n}}</math> <ref name="Häcker" /> | ||
| + | |||
| + | === NPV of a perpetuity === | ||
| + | Net cash flow is considered as perpetuity, when they occur constantly and infinitely. For this case, NPV can be expressed as follows <ref name="Häcker" />: | ||
| + | |||
| + | <math>N P V=-I_{0}+\frac{N C}{i}</math> <ref name="Häcker" /> | ||
== Applications == | == Applications == | ||
| − | + | ||
| + | For organizations, the NPV method helps decision-making in the investments of projects, programs, and portfolios since it helps to determine whether a given investment is profitable or loss-making. As explained in previous examples in the above sections, NPV can be calculated simply using the corresponding formulas. Generally, NPV is widely used to evaluate the financial appraisal of different types of investments such as independent projects, mutually exclusive projects, contingency projects, etc <ref name="Bora" />. | ||
| + | |||
| + | === Independent projects === | ||
| + | |||
| + | An Independent project is a project where the acceptance or rejection does not depend on the acceptance or rejection of other projects. If the organization has the capacity to run several projects at once, in the case of independent projects, all profitable projects will be accepted and ranking is not really important <ref name="Bora" />. | ||
| + | |||
| + | '''Example 3:''' | ||
| + | |||
| + | A company has an opportunity to invest in the construction of a new production line and at the same time the reconstruction of a factory currently used to produce one of its products. Net cash flows of the two projects are as follows and assume that the discount rate is 10% for both projects. | ||
| + | |||
| + | {| class="wikitable" | ||
| + | |+ style="caption-side:bottom; color:black;"|''Table 4: Example to illustrate the NPV of two idependendent projects. Own creation.'' | ||
| + | |- | ||
| + | ! style="color:black" | | ||
| + | ! style="color:black" | Now | ||
| + | ! style="color:black" | Year 1 | ||
| + | ! style="color:black" | Year 2 | ||
| + | ! style="color:black" | Year 3 | ||
| + | ! style="color:black" | Year 4 | ||
| + | ! style="color:black" | Year 5 | ||
| + | ! style="color:black" | NPV | ||
| + | |- | ||
| + | |Construction of a new production line | ||
| + | | -$1,000,000 | ||
| + | | $75,000 | ||
| + | | $275,000 | ||
| + | | $450,000 | ||
| + | | $500,000 | ||
| + | | $525,000 | ||
| + | | $301,037 | ||
| + | |- | ||
| + | |Reconstruction of a factory | ||
| + | | -$1,000,000 | ||
| + | | $200,000 | ||
| + | | $275,000 | ||
| + | | $350,000 | ||
| + | | $400,000 | ||
| + | | $425,000 | ||
| + | | $209,148 | ||
| + | |- | ||
| + | |} | ||
| + | |||
| + | The company can accept the investment in both projects since both NPV > 0 and the company has the ability to invest in both. | ||
| + | |||
| + | === Mutually exclusive projects === | ||
| + | |||
| + | |||
| + | Investment projects are said to be mutually exclusive when the selection of one will exclude the acceptance of the other. In simple terms, the acceptance of one prevents the acceptance of an alternative proposal. Because two or more projects cannot be pursued simultaneously due to different constraints in projects, programs, or portfolios. In the case of mutually exclusive projects; NPV is a widely used method to select which project or program is important to invest and the one with the higher NPV should be selected <ref name="Häcker" /> <ref name="Bora" />. | ||
| + | |||
| + | '''Example 4:''' | ||
| + | |||
| + | A company has a place, which can be used to build a food manufacturing business or a rubber manufacturing plant. The company cannot implement both projects due to financial constraints and assume that the risk associated with both projects are the same. The expected net cash flows associated with the two projects are as follows and assume a 10% discount rate. | ||
| + | {| class="wikitable" | ||
| + | |+ style="caption-side:bottom; color:black;"|''Table 4: Example to illustrate the NPV of two mutually exclusive projects. Own creation. '' | ||
| + | |- | ||
| + | ! style="color:black" | | ||
| + | ! style="color:black" | Now | ||
| + | ! style="color:black" | Year 1 | ||
| + | ! style="color:black" | Year 2 | ||
| + | ! style="color:black" | Year 3 | ||
| + | ! style="color:black" | Year 4 | ||
| + | ! style="color:black" | Year 5 | ||
| + | ! style="color:black" | NPV | ||
| + | |- | ||
| + | |Food manufacturing business | ||
| + | | -$4,000,000 | ||
| + | | $600,000 | ||
| + | | $800,000 | ||
| + | | $1,000,000 | ||
| + | | $1,600,000 | ||
| + | | $2,000,000 | ||
| + | | $292,591 | ||
| + | |- | ||
| + | |Rubber manufacturing plant | ||
| + | | -$4,000,000 | ||
| + | | $800,000 | ||
| + | | $1,000,000 | ||
| + | | $1,100,000 | ||
| + | | $1,200,000 | ||
| + | | $2,000,000 | ||
| + | | $441,624 | ||
| + | |- | ||
| + | |} | ||
| + | |||
| + | Even though both projects give positive NPV, the company cannot invest in both since they are mutually exclusive. Therefore it has to select the project which gives the highest NPV. Hence, in this case, the rubber manufacturing plant can be selected. | ||
| + | |||
| + | === Contingent projects === | ||
| + | |||
| + | |||
| + | Contingent projects are dependent projects where the acceptance or rejection of one project affects the acceptance or rejection of one or more other projects <ref name="Bora" />. For Example, the decision to invest in a medical testing laboratory may depend on the decision to build a private hospital nearby. The cash inflows of the medical testing laboratory will be enhanced by the presence of a nearby hospital and vice versa. This enhancement of the cashflows from the contingent projects must consider when calculating the NPV and to get the right decision. | ||
== Limitations == | == Limitations == | ||
| − | + | * NPV calculation is based on several estimates such as future cash flows and discount rate, therefore there is a high chance for errors that can drastically affect the end result of the calculation. Since every project has a unique set of processes there is lots of room for errors in estimating cash flows <ref name="ISO_21502"/>. NPV often takes an optimistic approach to future cash flow calculations by overestimating benefits (incoming cashflows) and underestimating costs (outgoing cashflows). These problems can be mitigated by double-checking estimates, performing sensitivity analysis or Monte Carlo analysis after doing the initial calculations <ref name="LOCK" /> <ref name="Ferrari"/>. On the other hand, Investment decisions based on the NPV method are highly sensitive to the selected discount rate <ref name="Ferrari"/>. Using a discount rate that is too low will make suboptimal investments and using too high will result in rejecting good investments. Moreover, the NPV is calculated based on the assumption that the discount rate remains the same throughout the life of the project. Therefore, it does not account for the fluctuation of discount rates according to the market situation <ref name="Bora" />. To remove this limitation NPV can be calculated using variable discount rates if they are known for the duration of the investment <ref name="Häcker" />. | |
| − | = | + | * The NPV is expressed in absolute terms rather than relative terms. Therefore it does not useful for comparing projects of different sizes as the largest projects typically generate the highest returns <ref name="Bora" />. If there are two projects for decision, and one project is larger in scale, the NPV will be higher for that project. Therefore, it is important to assess the returns from an investment in percentage terms to get an accurate picture of which investment provides a better return. |
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| + | * It does not consider the non-cash benefits and costs generated from a project while making decisions. NPV method is purely quantitive in nature and does not consider qualitative factors. However, it is important to consider both quantitative and qualitative benefits and costs associated with a project when taking the final decisions <ref name="ISO_21502"/>. | ||
| − | == Annotated Bibliography == | + | == Annotated Bibliography == |
| + | '''Häcker J. and Ernst D., Ch. 8 - Investment Appraisal. In: Financial Modeling. Global Financial Markets (2017), pp.343-384''' | ||
| − | ''' | + | The investment appraisal chapter in the financial modeling book by Häcker J. and Ernst D. introduces the concept of financial appraisal and provides a good overview of methods used in appraising a project, program, or portfolio. Writers introduce the concept of NPV and clearly describe the NPV calculation method and the decision rule behind the selection of projects based on NPV. This book is an ideal source to provide a good explanation of the concept of financial appraisal, the concept of NPV, how to calculate NPV, and other formulas of NPV in different scenarios such as annuity, perpetuity, etc. |
| + | |||
| + | '''Ferrari, C., Bottasso, A., Conti, M. and Tei, A., Ch. 5 - Investment Appraisal. In: Economic role of transport infrastructure: Theory and models (2018), pp. 85-114''' | ||
| + | |||
| + | Chapter 5 of this book introduces the different financial appraisal methods and it especially highlights the importance of NPV. This discusses the relationship of discount rate with NPV and the importance of selecting the correct discount rate when the projects are appraised based on NPV. Additionally, it introduces the importance of performing sensitivity analysis when calculating NPV. | ||
| + | |||
| + | '''LOCK, D., Project management. 10th edition, (2013)''' | ||
| + | |||
| + | This book discusses the importance of financial appraisal and business case development in projects, programs, and portfolios. This introduces the concept of NPV and its calculation method and highlights the significance of performing Monte Carlo analysis and sensitivity analysis. | ||
| + | |||
| + | '''Žižlavský O., ''Net present value approach: method for economic assessment of innovation projects., 19th International Scientific Conference, Economics and Management, (2014), pp. 506-512''' | ||
| + | |||
| + | The paper 'Net present value approach' by Žižlavský O. is a useful reference to get a thorough idea about the appraisal of projects using the NPV method. It highlights the importance of the time value of money and approaches used to select the suitable discount rate. | ||
| + | |||
| + | '''Bora, B., 'Comparison between net present value and internal rate of return', International Journal of Research in Finance and Marketing, (2015), pp.61-71''' | ||
| + | |||
| + | The research paper, 'comparison between net present value and internal rate of return' by Bora, B. provides a comprehensive discussion on the NPV method. This critically highlights the benefits and limitations of the NPV method and its applications in different investments. | ||
== References == | == References == | ||
<references> | <references> | ||
| − | <ref name="Häcker">Häcker J. and Ernst D., ''Ch. 8 - Investment Appraisal. In: Financial Modeling. Global Financial Markets | + | <ref name="Häcker">Häcker J. and Ernst D., ''Ch. 8 - Investment Appraisal. In: Financial Modeling. Global Financial Markets'', (Palgrave Macmillan, London, 2017), pp. 343-384, https://doi.org/10.1057/978-1-137-42658-1_8</ref> |
| − | <ref name="Ferrari">Ferrari, C., Bottasso, A., Conti, M. and Tei, A., ''Ch. 5 - Investment Appraisal. In: Economic role of transport infrastructure: Theory and models | + | <ref name="Ferrari">Ferrari, C., Bottasso, A., Conti, M. and Tei, A., ''Ch. 5 - Investment Appraisal. In: Economic role of transport infrastructure: Theory and models'', (Elsevier, 2018), pp. 85-114, https://doi.org/10.1016/C2016-0-03558-1</ref> |
| − | <ref name="Konstantin">Konstantin P. and Konstantin M., ''Ch. 4 - Investment Appraisal Methods. In: Power and Energy Systems Engineering Economics | + | <ref name="Konstantin">Konstantin P. and Konstantin M., ''Ch. 4 - Investment Appraisal Methods. In: Power and Energy Systems Engineering Economics'', (Springer, Cham, 2018), pp. 39-64, https://doi.org/10.1007/978-3-319-72383-9_4</ref> |
| − | <ref name="Poggensee">Poggensee K. and Poggensee J., ''Ch. 3 - Dynamic Investment Calculation Methods. In: Investment Valuation and Appraisal | + | <ref name="Poggensee">Poggensee K. and Poggensee J., ''Ch. 3 - Dynamic Investment Calculation Methods. In: Investment Valuation and Appraisal'', (Springer, Cham, 2021), pp. 85-140, https://doi.org/10.1007/978-3-030-62440-8</ref> |
| − | <ref name="Ing">Ing E. and Lester A., ''Ch. 6 - Investment Appraisal. In: Project Management, Planning and Control | + | <ref name="Ing">Ing E. and Lester A., ''Ch. 5 - Business case and Ch. 6 - Investment Appraisal. In: Project Management, Planning and Control'', 7th Edition, (Elsevier, 2017), pp. 25-36, https://doi.org/10.1016/B978-0-08-102020-3.00006-1</ref> |
| + | <ref name="Bora">Bora, B., ''Comparison between net present value and internal rate of return'', International Journal of Research in Finance and Marketing, (2015), 5(12), pp.61-71</ref> | ||
| + | <ref name="LOCK">LOCK, D., ''Project management'', 10th Edition, (Farnham, Surrey, England, Gower Pub., 2013).</ref> | ||
| + | <ref name="Žižlavský">Žižlavský, O., ''Net present value approach: method for economic assessment of innovation projects. Procedia-Social and Behavioral Sciences'', 19th International Scientific Conference, Economics and Management, 156 (2014), pp. 506-512.</ref> | ||
| + | <ref name="PRINCE2">AXELOS, ''Managing Successful Projects with PRINCE2'', 6th Edition, (The Stationery Office Ltd, 2017).</ref> | ||
| + | <ref name="PRINCE2_program">AXELOS, and Office Cabinet., ''Managing Successful Programmes'', 2011 Edition, (The Stationery Office Ltd, 2011).</ref> | ||
| + | <ref name="PRINCE2_portfolio">AXELOS, Office of Government Commerce, Kilford, C., ''Management of Portfolios'', 2011 Edition, (The Stationery Office Ltd, 2011).</ref> | ||
| + | <ref name="ISO_21502">International Organization for Standardization (ISO), ''Project, programme and portfolio management – Context and concepts (DS/ISO 21502:2020)'', 2021 Edition.</ref> | ||
| + | |||
</references> | </references> | ||
Latest revision as of 18:37, 6 April 2023
Written by Deepthi Tharaka Parana Liyanage Don- s203116
Contents |
[edit] Abstract
The financial appraisal is a method used to evaluate whether a proposed project, program, or portfolio is worthwhile by considering the benefits and costs that result from its execution [1]. Investment decisions of the management are critical to a company since it decides the future of the company [2]. This article discusses the net present value (NPV) method which is widely used in financial appraisal. NPV is a dynamic financial appraisal method that considers the time value of money by applying discounting to all future payments during the investment period [1]. In simple terms, NPV is the difference between the project's future incoming and outgoing cash flows at present time. NPV calculation begins with determining the net cash flows from the difference of future incoming and outgoing cash flows for each period of an investment. Once the net cash flow is calculated for each period, the present value (PV) is obtained by discounting using a suitable discount rate. NPV is the cumulative of all the present values of the future net cash flows [1] [3].
Firstly, this article discusses the idea behind the NPV method. Then this introduces the NPV calculation method and describes the importance of choosing the suitable discount rate. Then it highlights the decision criteria behind NPV and explains it with real-life applications. Also, it discusses the other NPV formulas for special cases such as annuity and perpetuity. Finally, it critically reflects on the limitations of this method and briefly highlights the key references used in this article.
[edit] Big idea
According to ISO 21502, a business case is a documented justification to support decision-making about the commitment to a project, program or portfolio [4]. Development of a business case is a critical point in a project, program or portfolio where it uses to obtain the approval by presenting the benefits, cost, and risk associated with alternative options [5] [6] [7]. The business case is produced by the client or the sponsor of the project, program or portfolio and the directorate of an organization uses this document to accept or reject the case. [8]. In a business case, financial appraisal plays a key role to answer the main questions of whether an investment should be made and which project should be chosen among a selection of different alternatives. Because the task of financial appraisal is to predict the financial effects of planned investment and to present the data to get critical investment decisions [1]. NPV method is one of the most frequently used approaches in the financial appraisal of a project, program, or portfolio [1] [9].
[edit] What is NPV?
NPV is the present value of the future net cash flows of an investment. [1]. In other words, NPV is the difference between the present value of all future incoming and outgoing cash flows. The NPV of a project is calculated based on different assumptions such as [10] [11] [12] :
- Money in the future will not be worth the same amount of money today. Therefore, all the future cash flows are converted to present value by using a suitable discount rate.
- The discount rate is constant throughout the lifetime of the project.
- The cash obtained by a project is reinvested immediately by the discount rate.
- All the incoming and outgoing cash flows except the initial investment occur at the end of each period.
NPV is a widely used method in the financial appraisal that has the following benefits [11] :
- NPV considers the concept of the time value of money by obtaining the present value of future net cash flows.
- NPV calculation considers the complete life cycle of an investment using all the future cash flows.
- It has the additive property. That means the possibility to sum the NPV of individual projects to evaluate the project portfolio.
- For businesses, the NPV method facilitates the decision-making process by identifying whether a project, program, or portfolio is profit-making or loss-making.
[edit] Time value of money
NPV is one of the most precise financial appraisal methods since it considers the time value of money in the analysis. Time value of money means that a sum of money is worth more now than the same sum of money in the future [2] [10] [11] . That is because the money you have now can use to make more money by buying something now and selling it later for more money, running a business, or depositing the money in the bank and earning interest. Since money can grow only through investing, investment delay is an opportunity cost. That means not having the money right now also includes the loss of additional income. Future money is also less valuable because inflation erodes its buying power. Moreover, receiving money in the future rather than now may involve some risk and uncertainty regarding its recovery. For these reasons, future cash flows are worth less than the present cash flows. NPV assesses the profitability of a given project on the basis that cash flow in the future is not worth the same as a cash flow today. The time value of money is recognized in NPV by applying discounting to all future cash flows generated during the investment period using a suitable discount rate[9].
[edit] Discount rate
The discount rate is the rate used to determine the present value of future cash flows in NPV calculation. The choice of the discount rate can dramatically change the NPV since the NPV is an inverse function of the discount rate (i) [3]. The selection of discount rate will be company-specific since it is related to how the company finds its funds [10]. If an investment is financed completely with equity, the discount rate can be obtained from a cost of equity. On the other hand, if the investment is completely debt-financed it can be analyzed using the cost of debt. If an investment depends on both equity and debt financing, the discount rate is the weighted average cost of capital (WACC) and can be calculated as the weighted average of the cost of equity and cost of debt [10]. WACC is often used in companies as the discount rate [1]. For small projects, where it is hard to identify the equity and debt used for financing a single project, the WACC of the whole company is usually assumed as the discount rate [10].
However, it is appropriate to use higher discount rates to reflect other factors such as risk, the opportunity cost of money invested in the infrastructure and etc [3]. Also, NPV calculated using variable discount rates may correctly interpret the situation than one calculated from a constant discount rate. [1].
[edit] How to calculate NPV?
The following formulas use these common variables [1]:
| Variable | Meaning |
|---|---|

|
Present value |
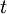
|
Time of the cashflow |
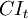
|
Cash inflows in period t |
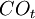
|
Cash outflows in period t |
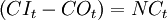
|
Net cash flow in period t |
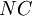
|
Constant net cash flow for all periods |
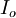
|
Investment in t=0 |
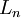
|
Liquidation proceeds in t=n |
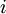
|
The discount rate |
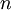
|
Total number of periods |
In order to consider the time value of money, the NPV method considers the value of an investment at the present time by projecting all future cash flows into a key figure at the time today. This projection of the value that a future cash flow has in the present is called present value (PV). To get the present value of a future net cash flow in period t, it is discounted using a discount rate (i) as follows:
Example 1:
If a project is expected to receive a net cash flow of $5,000 with 10% discount rate after 4 years, its present value can be calculated as:

NPV combines these projections of all net cash flows to assess the overall value of a project from the present view. Therefore the NPV is given by:

The difference between the cash inflows and cash outflows,  gives the net cash flow,
gives the net cash flow, 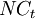 of the corresponding period. For investments which are characterized by initial investment,
of the corresponding period. For investments which are characterized by initial investment, 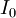 , subtracting it from the present value of the net cash flows gives the net present value. If a possible final payment,
, subtracting it from the present value of the net cash flows gives the net present value. If a possible final payment, 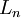 , for the liquidation of the investment in t = n is also considered, the NPV can be calculated as follows [1]:
, for the liquidation of the investment in t = n is also considered, the NPV can be calculated as follows [1]:
As a summary, the following steps are recommended for the calculation of the net present value [1]:
- Determination of the initial investment,
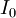 .
.
- Estimate the expected net cash flows,
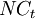 for each period.
for each period.
- Selection of the constant discount rate,
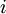 .
.
- Determination of the present values by discounting the net cash flows and finally obtaining the summation.
- Subtraction of the initial investment,
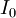 from the summation of the present values. This gives the net present value.
from the summation of the present values. This gives the net present value.
Example 2:
A company decides to invest $100,000 for a glass manufacturing plant which is expected to give the following cash flows. Assume the discount rate is 10%. Calculate the NPV of the project.
| Now | Year 1 | Year 2 | Year 3 | |
|---|---|---|---|---|
| Initial Investment | $100,000 | |||
| Running costs | $60,000 | $90,000 | $90,000 | |
| Revenues | $80,000 | $100,000 | $120,000 | |
| Liquidation proceeds | $140,000 |
NPV can be calculated using the formula as follows.
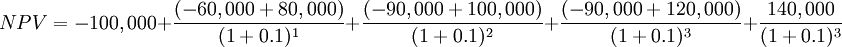
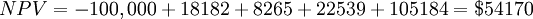
[edit] Decision rule
NPV is used to assess the attractiveness of the investment and it shows how much value an investment or project adds to the firm and the decision to accept or reject the investment is taken based on the following decision rule [1].
| Condition | Idea | Decision |
|---|---|---|
| If NPV > 0 | Investment increases the value (profit) of the company in the amount of the NPV. | Investment can be accepted. |
| If NPV = 0 | No increase or decrease in value of the company. | This project adds no monetary value. Difficult to get the decision whether to accept or reject the project. Decisions should be based on other criteria. |
| If NPV < 0 | Investment would decrease value (profit) in an amount equal to the negative NPV. | The investment can be rejected. |
As a summary, the following two profitability criteria must be met for the acceptance of an investment [1]:
- Absolute profitability: NPV of an investment option must be positive or at least zero (NPV≥ 0).
- Relative profitability: If there are several positive NPV options, the one with the highest NPV should be selected.
[edit] Other NPV formulas for special scenarios
Note that this section also uses the same variables as listed in Table 1 above.
[edit] NPV of an annuity
Net cash flows can be interpreted as an annuity, if they are constant, equidistant and occur at the end of each period [1]. Using the formula for the present value of an ordinary annuity, NPV can be calculated as follows [1]:
[edit] NPV of a perpetuity
Net cash flow is considered as perpetuity, when they occur constantly and infinitely. For this case, NPV can be expressed as follows [1]:
[edit] Applications
For organizations, the NPV method helps decision-making in the investments of projects, programs, and portfolios since it helps to determine whether a given investment is profitable or loss-making. As explained in previous examples in the above sections, NPV can be calculated simply using the corresponding formulas. Generally, NPV is widely used to evaluate the financial appraisal of different types of investments such as independent projects, mutually exclusive projects, contingency projects, etc [11].
[edit] Independent projects
An Independent project is a project where the acceptance or rejection does not depend on the acceptance or rejection of other projects. If the organization has the capacity to run several projects at once, in the case of independent projects, all profitable projects will be accepted and ranking is not really important [11].
Example 3:
A company has an opportunity to invest in the construction of a new production line and at the same time the reconstruction of a factory currently used to produce one of its products. Net cash flows of the two projects are as follows and assume that the discount rate is 10% for both projects.
| Now | Year 1 | Year 2 | Year 3 | Year 4 | Year 5 | NPV | |
|---|---|---|---|---|---|---|---|
| Construction of a new production line | -$1,000,000 | $75,000 | $275,000 | $450,000 | $500,000 | $525,000 | $301,037 |
| Reconstruction of a factory | -$1,000,000 | $200,000 | $275,000 | $350,000 | $400,000 | $425,000 | $209,148 |
The company can accept the investment in both projects since both NPV > 0 and the company has the ability to invest in both.
[edit] Mutually exclusive projects
Investment projects are said to be mutually exclusive when the selection of one will exclude the acceptance of the other. In simple terms, the acceptance of one prevents the acceptance of an alternative proposal. Because two or more projects cannot be pursued simultaneously due to different constraints in projects, programs, or portfolios. In the case of mutually exclusive projects; NPV is a widely used method to select which project or program is important to invest and the one with the higher NPV should be selected [1] [11].
Example 4:
A company has a place, which can be used to build a food manufacturing business or a rubber manufacturing plant. The company cannot implement both projects due to financial constraints and assume that the risk associated with both projects are the same. The expected net cash flows associated with the two projects are as follows and assume a 10% discount rate.
| Now | Year 1 | Year 2 | Year 3 | Year 4 | Year 5 | NPV | |
|---|---|---|---|---|---|---|---|
| Food manufacturing business | -$4,000,000 | $600,000 | $800,000 | $1,000,000 | $1,600,000 | $2,000,000 | $292,591 |
| Rubber manufacturing plant | -$4,000,000 | $800,000 | $1,000,000 | $1,100,000 | $1,200,000 | $2,000,000 | $441,624 |
Even though both projects give positive NPV, the company cannot invest in both since they are mutually exclusive. Therefore it has to select the project which gives the highest NPV. Hence, in this case, the rubber manufacturing plant can be selected.
[edit] Contingent projects
Contingent projects are dependent projects where the acceptance or rejection of one project affects the acceptance or rejection of one or more other projects [11]. For Example, the decision to invest in a medical testing laboratory may depend on the decision to build a private hospital nearby. The cash inflows of the medical testing laboratory will be enhanced by the presence of a nearby hospital and vice versa. This enhancement of the cashflows from the contingent projects must consider when calculating the NPV and to get the right decision.
[edit] Limitations
- NPV calculation is based on several estimates such as future cash flows and discount rate, therefore there is a high chance for errors that can drastically affect the end result of the calculation. Since every project has a unique set of processes there is lots of room for errors in estimating cash flows [4]. NPV often takes an optimistic approach to future cash flow calculations by overestimating benefits (incoming cashflows) and underestimating costs (outgoing cashflows). These problems can be mitigated by double-checking estimates, performing sensitivity analysis or Monte Carlo analysis after doing the initial calculations [2] [3]. On the other hand, Investment decisions based on the NPV method are highly sensitive to the selected discount rate [3]. Using a discount rate that is too low will make suboptimal investments and using too high will result in rejecting good investments. Moreover, the NPV is calculated based on the assumption that the discount rate remains the same throughout the life of the project. Therefore, it does not account for the fluctuation of discount rates according to the market situation [11]. To remove this limitation NPV can be calculated using variable discount rates if they are known for the duration of the investment [1].
- The NPV is expressed in absolute terms rather than relative terms. Therefore it does not useful for comparing projects of different sizes as the largest projects typically generate the highest returns [11]. If there are two projects for decision, and one project is larger in scale, the NPV will be higher for that project. Therefore, it is important to assess the returns from an investment in percentage terms to get an accurate picture of which investment provides a better return.
- It does not consider the non-cash benefits and costs generated from a project while making decisions. NPV method is purely quantitive in nature and does not consider qualitative factors. However, it is important to consider both quantitative and qualitative benefits and costs associated with a project when taking the final decisions [4].
[edit] Annotated Bibliography
Häcker J. and Ernst D., Ch. 8 - Investment Appraisal. In: Financial Modeling. Global Financial Markets (2017), pp.343-384
The investment appraisal chapter in the financial modeling book by Häcker J. and Ernst D. introduces the concept of financial appraisal and provides a good overview of methods used in appraising a project, program, or portfolio. Writers introduce the concept of NPV and clearly describe the NPV calculation method and the decision rule behind the selection of projects based on NPV. This book is an ideal source to provide a good explanation of the concept of financial appraisal, the concept of NPV, how to calculate NPV, and other formulas of NPV in different scenarios such as annuity, perpetuity, etc.
Ferrari, C., Bottasso, A., Conti, M. and Tei, A., Ch. 5 - Investment Appraisal. In: Economic role of transport infrastructure: Theory and models (2018), pp. 85-114
Chapter 5 of this book introduces the different financial appraisal methods and it especially highlights the importance of NPV. This discusses the relationship of discount rate with NPV and the importance of selecting the correct discount rate when the projects are appraised based on NPV. Additionally, it introduces the importance of performing sensitivity analysis when calculating NPV.
LOCK, D., Project management. 10th edition, (2013)
This book discusses the importance of financial appraisal and business case development in projects, programs, and portfolios. This introduces the concept of NPV and its calculation method and highlights the significance of performing Monte Carlo analysis and sensitivity analysis.
Žižlavský O., Net present value approach: method for economic assessment of innovation projects., 19th International Scientific Conference, Economics and Management, (2014), pp. 506-512
The paper 'Net present value approach' by Žižlavský O. is a useful reference to get a thorough idea about the appraisal of projects using the NPV method. It highlights the importance of the time value of money and approaches used to select the suitable discount rate.
Bora, B., 'Comparison between net present value and internal rate of return', International Journal of Research in Finance and Marketing, (2015), pp.61-71
The research paper, 'comparison between net present value and internal rate of return' by Bora, B. provides a comprehensive discussion on the NPV method. This critically highlights the benefits and limitations of the NPV method and its applications in different investments.
[edit] References
- ↑ 1.00 1.01 1.02 1.03 1.04 1.05 1.06 1.07 1.08 1.09 1.10 1.11 1.12 1.13 1.14 1.15 1.16 1.17 1.18 1.19 1.20 1.21 1.22 1.23 1.24 1.25 1.26 Häcker J. and Ernst D., Ch. 8 - Investment Appraisal. In: Financial Modeling. Global Financial Markets, (Palgrave Macmillan, London, 2017), pp. 343-384, https://doi.org/10.1057/978-1-137-42658-1_8
- ↑ 2.0 2.1 2.2 LOCK, D., Project management, 10th Edition, (Farnham, Surrey, England, Gower Pub., 2013).
- ↑ 3.0 3.1 3.2 3.3 3.4 3.5 Ferrari, C., Bottasso, A., Conti, M. and Tei, A., Ch. 5 - Investment Appraisal. In: Economic role of transport infrastructure: Theory and models, (Elsevier, 2018), pp. 85-114, https://doi.org/10.1016/C2016-0-03558-1
- ↑ 4.0 4.1 4.2 International Organization for Standardization (ISO), Project, programme and portfolio management – Context and concepts (DS/ISO 21502:2020), 2021 Edition.
- ↑ AXELOS, Managing Successful Projects with PRINCE2, 6th Edition, (The Stationery Office Ltd, 2017).
- ↑ AXELOS, and Office Cabinet., Managing Successful Programmes, 2011 Edition, (The Stationery Office Ltd, 2011).
- ↑ AXELOS, Office of Government Commerce, Kilford, C., Management of Portfolios, 2011 Edition, (The Stationery Office Ltd, 2011).
- ↑ Ing E. and Lester A., Ch. 5 - Business case and Ch. 6 - Investment Appraisal. In: Project Management, Planning and Control, 7th Edition, (Elsevier, 2017), pp. 25-36, https://doi.org/10.1016/B978-0-08-102020-3.00006-1
- ↑ 9.0 9.1 Konstantin P. and Konstantin M., Ch. 4 - Investment Appraisal Methods. In: Power and Energy Systems Engineering Economics, (Springer, Cham, 2018), pp. 39-64, https://doi.org/10.1007/978-3-319-72383-9_4
- ↑ 10.0 10.1 10.2 10.3 10.4 Žižlavský, O., Net present value approach: method for economic assessment of innovation projects. Procedia-Social and Behavioral Sciences, 19th International Scientific Conference, Economics and Management, 156 (2014), pp. 506-512.
- ↑ 11.0 11.1 11.2 11.3 11.4 11.5 11.6 11.7 11.8 Bora, B., Comparison between net present value and internal rate of return, International Journal of Research in Finance and Marketing, (2015), 5(12), pp.61-71
- ↑ 12.0 12.1 12.2 Poggensee K. and Poggensee J., Ch. 3 - Dynamic Investment Calculation Methods. In: Investment Valuation and Appraisal, (Springer, Cham, 2021), pp. 85-140, https://doi.org/10.1007/978-3-030-62440-8
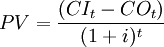
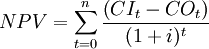
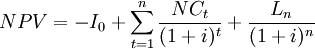
![N P V=-I_{0}+N C \cdot\left[\frac{(1+i)^{n}-1}{i \cdot(1+i)^{n}}\right]+\frac{L_{n}}{(1+i)^{n}}](/images/math/d/c/0/dc008a1c76fe4715376d686b725c83a9.png)