Revision as of 14:38, 26 September 2015

Introduction

In order to advance, management, and more specifically strategic management, needs to integrate other scientific disciplines in its own concept. Game theory is an aspect of mathematics designed to understand, model and predict the behaviour of actors considering a determined environment. Basically, the theory identifies the players, their assets and their possible strategies. It simulates, through a precise defined game, the different interactions between entities such as companies, states, lobbies, individuals etc. The concept is already widely used in many fields such as psychology, economics, politics and others. Despite its great efficiency, some prerequisites of Game theory may seem contradictory with a management point of view. First, the extreme mathematical complexity needed to treat some problems is an important limitation. Secondly, the presumed rationality of the players can be defected in the real world.

Regarding some simple games, such as the prisoner´s dilemma, this article focuses on the possible outcomes of Game theory leading to development of models and decision making process in management. Going through different scenarios and examples while considering the ethical point of view, this article outlines the use of Game theory as a simple tool. It opens a new and more rational perspective for the manager that can be used in addition to usual managerial skills.

The concept

Overview

Game theory is, at first, a mathematic discipline based on a very high formal demand. This article will not try to explain this aspect. Therefore it has to be considered only as an introduction of the concept of game theory and its possible outcomes regarding project management. Here ^[1] the lector will be able to find a more formal presentation of Game theory.

Game theory 's main goal is to help to achieve successful decision making process. In order to achieve these objectives, the situation has to be studied in a very precise and special framework. This framework is destined to modulate a game, the more the situation can easily be assimilated as such, the more the theory will be effective. The translation from a real world situation to a game is done through high mathematical formalism. Moreover, the framework also requires to identify the payoff each player is looking for and a set of rules. Most of the rules can be determined regarding the situation, others are inherent to game theory, that is:

- The players act rationally.

- The players act egoistically.

The target is always to optimize one’s own payoff. To do so, it is however important that the player do not only consider the different strategies available to himself, but also anticipates the optimal strategy of the other players in order to predict their choices. In most of the games, the payoff depends on strategies of others and therefore the concept of reciprocal influence plays a huge part in the way the game is played. For a complete presentation of the different terms used in Game theory : ^[2]

Different types of games can be identified regarding the basic settings. For example, games can be symmetric, meaning that both players start the game with equal settings and possibilities, or asymmetric meaning that the choice of the same strategies will not lead to the same outcomes for the players. One can be considered as having an advantage.

Games can also be cooperative: the players can create alliances and communicate. It is also possible to decide if players are aware of what the others are doing or not to simulate real-life situation.

Finally there are zero-sum games where each players act alone regarding its own best interest. The payoffs are fixed to a certain value, and a player winning means that others have to lose. It is possible to consider a cake cut in different parts to illustrate the idea. If one player takes a big part, then the others will automatically get less payoff i.e. less cake regarding the example. No cooperation or alliances can ever change this outcome, the game is defined as being strictly competitive.

In opposite is non-zero-sum game, gains from one player does not imply losses for another one. The amount of gain possible is not fixed.

First example : The prisoner's dilemma

The prisoner's dilemma is one of the most famous game in Game theory .

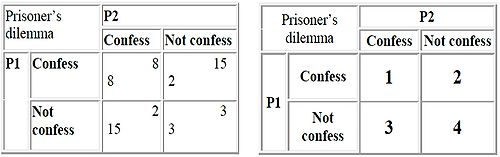
It consists in a simple non-zero-sum and non-cooperative game. Players cannot communicate. Background of the game: 2 people have been arrested. The evidence ensure they will get at least 3 years of prison even if they do not confess. They want to minimize the amount of prison years they will get. The following table shows a visual representation of the game:

Figure 1: Prisoner dilemma

The table is important to understood. This example will be used to explain some general notion of game theory:

The dominant strategy: the best strategy regarding the payoff and regardless what the others players are doing. First, it is important to notice that the prisoner’s dilemma is a symmetric game. We can now consider Player 1: if he confesses and P2 Confesses P1 gets 8 years, if he does not confess and P2 confesses P1 takes 15 years, so confessing is better.

Again if P1 confesses and P2 does not P1 takes 2 years and if P1 does not confess and P2 does not confess P1 takes 3 years so confessing is better.

Regardless what Player 2 is doing, confessing gives the better outcome for P1, therefore it is the dominant strategy.

The Nash equilibrium: A Nash equilibrium is defined as a solution where no players can increase its payoff by moving away from it.

The prisoner’s dilemma can be used again:

We can start with the combination 2 (P1 confess and P2 doesn’t). Considering that P2 doesn’t confess, should P1 not confess? No, he will take 3 years instead of 2. On the other hand P2 should change his choice i.e. confess in order to get 8 years instead of 15, therefore it is not a Nash equilibrium.

In combination 4, both players can increase their outcome (reducing the amount of years in prison) by confessing so this is not a Nash equilibrium either. Combination 3 is symmetric to 2.

The dominant strategy, 1: if one of the two players decide to change his strategy, he will take 15 years instead of 8 years. Therefore there is no reason to change and in this particular case the dominant strategy is also a Nash equilibrium.

Then, it appears that the outcomes 2, 3 and 4 should never happened if the players play regarding their own best interest. Of course one can immediately notice that the combination 4 will be better for both players. But regarding the rules (no communication and no information about what the others players is doing), the only possible outcome is 1 (the players acting rationally and egoistically).

The prisoner’s dilemma is a basic game, however it is really useful to understand the concept of the theory and its possible applications regarding decision-making.

The complexity of modelling real life situation through game theory is virtually unlimited. However the mathematical formalisation become more and more complex at the same time. In theory, it is possible to model games with infinite players and set as many rules as needed in order to represent reality: the game can be sequential or non-sequential, the information available can be complete or not, players can be able to communicate, to form coalition…

Application and use

The need to take strategic behaviour into account

Strategic behaviour should be taken into account when the stakes are high. If Game theory 's outcomes are not always shaped to reality, then people thinking through Game theory ’s basis will not act as we could expect or desire in reality. Therefore one should always take into account the possibility of pure strategic behaviour from one or several elements. Then, a manager have to be aware of this different way of thinking in order to choose and enforce the best strategy to achieve its goals.

For one example when organizers have failed to implement effective rules, we can consider the London Olympics in 2012 and more specifically the badminton teams.

The tournament consists in 2 phases:

Phase 1: 16 teams, 4 groups of 4 teams and the best 2 teams of each group advanced.

Phase 2: 8 teams, immediate elimination.

What creates the issue is that the Danish team made a huge upset defeating one of the Chinese team WC during Phase 1, considered to be by far the strongest one. Therefore QW advanced 2nd of its group to the phase 2. It influenced the bracket of Phase 2. The problem can be defined as a misalignment between what the Committee desired to create and what the athletes wanted. The Committee wanted great and fair games, the athletes wanted to win the best medal possible.

After the defeat of QW, it happens that in the last day of Phase 1, two others matches go wrong. In both matches, the winner would meet QW in semi-final, whereas the loser would only meet them in final in the Phase 2 of the tournament (all the teams would reach Phase 2 no matter the issue of these specific matches). So if the teams mentionned lost their last match in Phase 1, they will still advanced in Phase 2 and they will also meet the strongest team later. Considering these facts, all 4 teams in both matches tried their best to…lose.

From a game theory point of view, the behaviour of the teams is perfectly logical and understandable. They chose their strategy, in this case losing, in order to optimized their possible outcomes (getting the best medal possible) and taking into account the frame of the game (meaning they tried to meet QW the latest possible, the team being considered as unbeatable).

Here is a short video showing a part of one of the matches. Keep in mind: if the team loses, they go to phase 2 and meet QW only in the finals meaning they have a great chance to get to silver medal.

Even if the comportment of the players can be put into question (indeed, the teams have been excluded from the tournament) they never broke any explicit rules. Actually one could say they act rationally and egoistically. This example is meant to show that strategic behaviour can be used at all levels. Therefore a manager should at least be aware of the possible strategies available for the different stakeholders he is dealing with during a project. Moreover he could himself use some outcomes of Game Theory.

Agreement game

From a managerial point of view, Game theory is a very good tool to get into other people shoes and try to fully understand their point of view and their options. With a game theory vocabulary, the main goal is to fully understand all the strategies available for all the players. Then the model can help to choose the best strategy in order to maximise one’s own profit, or a coalition profit, or just chose the best reply in order to defeat the opponent in zero-sum game.

However some situations can be directly treated trough game theory. The following presents some simples examples where game theory can model a management issue and bring a satisfying solution.

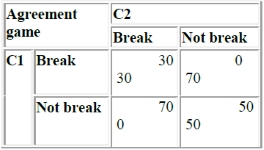
For example an agreement between 2 companies can be modelled considering a precise market:

Company 1, C1, and company 2, C2, agreed to share a market and release a certain amount of a product P in order to keep the price high. If C1 and C2 keep the agreement they sell P at 50. If one breaks the agreement, we will assume that he will be able to win on the long term the entire market and therefore sell the product to a higher price: 70. If they both break the agreement and try to increase their sells, there will be an overproduction of P, the market will saturate and the price will decrease: 30. This game can be represented through a table:

Figure 2: Agreement game

It is interesting to see that the Nash equilibrium in this situation consists in C1 and C2 breaking the agreement. However it put them in a situation less favourable than keeping the agreement intact.

Nevertheless such a situation is instable because the possible outcome in breaking the agreement is really attractive. This situation will require an extra set of rules to maintain the most profitable outcome for both companies. In reality, it means the creation of an authority able to guarantee the respect of the agreement (contracts, etc….). Acknowledging the necessity of such authority can be very important regarding the first steps of a project or a program. It is a valuable information for a manager.

One can also simply consider that truth between the companies can be enough, but such a thing as truth does not exist according to the basics rules of Game Theory. And it has been shown that strategic behaviour can occur at all levels. We will consider this aspect more precisely at the end of this article.

Market entry game

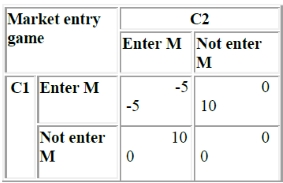
Keeping the model of the prisoner’s dilemma we can think of a game simulating the entry in the market of a new product.

Two companies, C1 and C2, share a market M. In a simple case, both companies sell the same product P, and will both be as good as selling it. The cost to develop P is 20.

M represents a total value of 30.

Therefore, if both enter the market at the same time, they will both lose money, the cost of production being higher than the expected benefit coming from the exploitation of M. If one does not enter the market he will not have any production cost, but also will not be able to make profit out of the product.

This is a simultaneous competitive game. We can model him through a table similar to the prisoner’s dilemma:

Figure 3: Market entry

It can easily be seen that the top right and the bottom left cases are both Nash equilibrium, meaning that both companies have no interest to change their strategies if a Nash equilibrium happens.

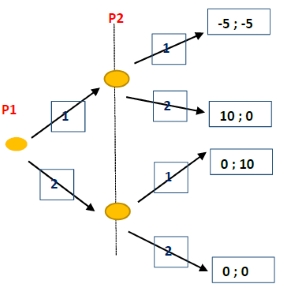
Through this model we can also consider another aspect of games, the first move advantage (FMA). If we consider this game as sequential (and therefore abandoned the simultaneous part), we can highlight the fact that the market entry game presents a FMA.

Notations:

• 1: enter the market
• 2: doesn't enter the market

In the figure the same game is represented as sequential, meaning one player chooses its strategy first and the others have to reply afterwards.

Figure 4: Sequential version

It is now clear that if P1 has the opportunity to make the first move, he will surely enter the market i.e. chose 1. Then P2 has no choice but to not enter the market in order to reduce its loss. P1 ends up with the best outcome possible; a benefit of 10. Recognizing a FMA in a situation is very important, some complex situations (during projects regarding negotiations or when some important choices have to be made)can make people too cautious or even passive. Waiting for someone else to unlock the situation, make the first move, can already be a failure.

Coalition game

Finally a more complicated game based on coalition will be presented. It suits better the environment of companies but also requires more formalism.

It consists in the development of a new cd format (BLU RAY for example) which will oppose to a former or competitive one: High Quality DVD.

In order to achieve a full product, a cd company constructor (hardware i.e. lectors) and editors (cd producers: movies, games) are needed. There are therefore constructors and editors.

We call consortium the association of constructors and editors who have chosen the same format i.e. the same product. In order to simplify, it will also be assumed that the size of the consortium has a direct impact on the benefit he may realize: the bigger, the stronger and the better.

In another hand the redistribution of the overall benefits inside the consortium will decrease with the number of participants i.e. with the size of the consortium. Therefore a consortium too important may not be wanted.

We define:

- N, the total amount of companies.

- n, the amount of companies in one consortium.

- T the total amount of benefit expectable in the entire market.

- B(x,y) the benefit of a consortium of x constructors and y editors . The other will have T-B.

- a the part of the market wins by one consortium (the other would have 1- a).

Therefore the Profit P realised by one constructor in a consortium is:

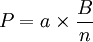
We will consider a low amount of companies (4 and 4) in order to simplify the formalism of the game. 1/1/1/1 means that all the companies (editors or constructors) have chosen the format 1.

Their benefits is therefore:

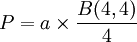
Then, regarding the different values and scenario, the possible evolution of the situation can be evaluated.

If one constructor decides to leave the consortium for the other format and manages to get an editor with him, then we have:

1/1/1/2 and two types of profit:

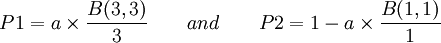
The situation is stable for the consortium 1 if 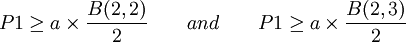etc…

For the consortium 2, the stability is given by:

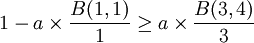
It means that the editor have a better outcome by staying with the constructor who have chosen 2, and the constructor gets more outcome that he had in the first consortium.

This example shows that Game theory is able to model complex interactions between companies as well as represent different types of real life situation such as the need to create alliances in order to survive. For a complete explanation of this example, please consult : ^[3]

Limitations

Never ending games: Game theory with no answers

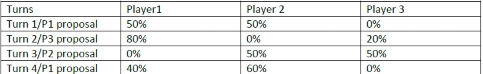
All real life situations can in theory be simulated, however Game theory is unable to provide valid solution for all of them. Imagine 3 people who want to share a cake(a market, parts of a project...). The decision process is democratic, if a majority agrees, the 3rd one have no choice.

1, 2 and 3 are the players. The table presents the possibility of a game, proposal by proposal.

Figure 5: Cake sharing

We rapidly see a cycle appears, all the strategies are dominated by others. The game will never end regarding game theory, because one player can always make a proposal which will suits better to two players. It appears here that Game theory cannot answer all types of situation. Only negotiations skills can allow one player to end the game.

Irrational concept of rationality

• The concept of rationality is also very often in contradiction with real life behaviour.

In fact, people often act irrationally and are not egoistic, at least at a small scale. This can be illustrate by the dictator game:

One player decide to give a percentage of a sum to another player, and this one has to accept (passive player).

Considering 100 the sum, the player should give 0 and keep 100. But studies have shown that most of the time people decide to give some money to the other player, often 40.

In another version of the game, the player 2 has to decide if he accepts the donation, or refuses. In the last case, no one gets money.

In Game theory , even a 99/1 repartition should be accepted, because player 2 would still optimize his outcome by accepting. However, these types of offers are very often rejected. It is therefore necessary to consider concept such are punishment, rewards, equity. These are very important in order to simulate human behaviour, but are not included in Game theory . In a project, winning the trust of others is important, being reasonably fair also is. Indeed, if a project (or at least some parts of it) can be modelled as a game, it is not completely over after the endgame, the completion of the project. The image the company has given, the relations with the stakeholders, often has an importance beyond the project, whereas Game theory only optimizes one situation regardless the other players and the possible future games to play.

Mathematical dimension

• Another limitation of Game theory already mentioned is the mathematical complexity that is rarely accessible for most.

Conclusion

Despite its inherent defaults, Game theory isn’t closed regarding the possible outcomes. If profit is often chosen as the target outcome, the stability of the solution can also be considered. Applied to huge entities, Game Theory becomes even more effective as we get generally closer to the rational and egoistic behaviour. Moreover, the construction of the model highlights all the possible outcomes regarding the different strategies. From this range of possibilities, the manager can then choose the most adequate solution based on a human knowledge of the situation, for example the necessity to be fair towards the “opponent”, who may be the client or an other company, even if it means reducing the payoff.

It is, at least, very important to be aware of such a way of modelling. Strategies out of Game Theory are in certain cases incredibly effective, surely because they do not take the human factor into account. This aspect is both the strength and the weakness of the concept, as every tools, it all depends on how to use it. The manager have to master the utilisation he is making out of it.

Reference and further readings

Websites

James Miller (2015): Introductory Game Theory Videos. These videos have been realised by James Miller, teacher at Smith Collegium. These resource should be used in order to complement further reading or courses on Game Theory and focuses on particular examples and notions. It requires knowledge and a certain background about Game Theory. However they are designed for students and should suit anyone who wants to acquire knowledge on the subject.

Ben Polak :Yale courses on Game Theory. These videos have been released according to Yale's program of knowledge's accessibility. It consists in real courses filmed at Yale University. Therefore, the first lessons are accessible to anyone and provide a great background and explanation if Game Theory. It is also a great way to approach step by step the mathematical view of Game Theory. However some prerequisites in mathematics are expected in order to fully profit from the courses.

Thomas S.Ferguson : Mathematical approach of Game Theory. The website consists on a great and formal explanation of particular aspects of Game Theory such as coalition or zero-sum games. The lector should be aware that the lecture will require some skills in Mathematics as well as a certain attraction to formal presentation ( theorems, demonstration, exercises). It is however a great way to go further into Game Theory's understanding.

Don Ross : Stanford encyclopedia article on Game Theory. This article published by the Stanford encyclopedia of Philosophy offers a great presentation of Game Theory as well as general reflexions on the subject. Therefore it can be a great way to discover the Theory. It also provides technical information while keeping low the mathematical aspect which is ideal if the lectors does not possess a strong mathematical background.

Text references

1. ↑ [http://www.math.ucla.edu/~tom/Game_Theory/Contents.html] Mathematical approach of Game Theory by Thomas S. Ferguson
2. ↑ [https://en.wikipedia.org/wiki/Game_theory] Wikipedia page on Game Theory
3. ↑ [1]

Printed references

Christian Julmi : Introduction to Game Theory, available on bookboon.com [2].

Nabyla DAIDJ and Abdelhakim HAMMOUDI : Paper on game theory entitled "Les apports de la théorie des jeux au management stratégique" [3].