Internal rate of return (IRR)

[Lorenzo Incarnato s220426]

- references - pictures

Abstract

The Internal Rate of Return (IRR) is a powerful discounted cash flow method used in capital budgeting and corporate finance to estimate and evaluate the profitability of potential investments. Keeping in mind the formula for the Net present value (NPV), the IRR is defined as the discount rate that makes the present value of the costs (negative cash flows) of an investment equal to the present value of the benefits (positive cash flows). In other words, the IRR is the discount rate that gives a net present value of zero when applied to the expected cash flow of a project. This rate of return is called internal because the formula predicts a rate that depends only on the project, more precisely on the project's cash flows, and does not depend on external factors such as market interest rates or inflation. One of the main advantages in using the internal rate of return to evaluate project investments, compared to other methods such as the Payback period or the Benefit-cost ratio, is that IRR considers the time value of money. In general, due to the relationship between NPV and IRR, the higher the Internal Rate of Return of a project, the more desirable the investment to be made. This article shows also the limitations of the internal rate of return; however, when the IRR is unique, it provides relevant information about the return on investment and is also used as a measure of investment efficiency. In fact, according to academic research, three-quarters of Chief Financial Officers use the IRR method to evaluate capital projects.

Time value of money

A key consideration regarding discounted cash flow methods is the Time Value of Money (TVM). This paragraph explains why money tomorrow is not as valuable as money today. In finance, an amount of money available today or in the future has a different value. In other words, $ 100 today is not $ 100 tomorrow and this difference is due to the time value of money.

The reason is that today you have the opportunity to invest the money and thus grow, according to the time horizon and the interest rate. Another reason for the time value of money is the purchasing power of money which changes over time due to inflation or deflation. Hence, the time has a monetary value and we have to consider it if we want to compare money available in different moments . It is, therefore, necessary to briefly underline three fundamental terms about investments to clearly understand the time value of money:

• The Present value PV: the sum of money available today that can be invested [$];
• The Interest rate (or opportunity cost if we decide not to invest) r: the amount of interest due per period, as a proportion of the amount deposited or borrowed [%]
• The Future value FV: is the value of a current asset (present value) at a future date based on an assumed rate of growth (interest rate) [$].

Present to Future	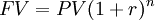$FV = PV (1 + r)^n$
Future to Present	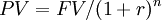$PV = FV / (1 + r)^n$

Example 1: Would you rather have $ 100,000 today or the same amount in 1 year from now? To calculate how much money will be $ 100,000 in a year from now, supposing an interest rate r = 3%, we can apply the formula for the future value:

$FV = PV * (1 + r)^n = $ 100,000 * (1 + 0.03)^1 = $ 103,000$

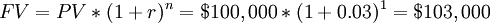
So having $ 100,000 now is equivalent to having $ 103,000 in the future considering an interest rate of 3%. If we agreed to receive $ 100,000 in one year instead of now, we would be $ 3,000 less. As shown, the present value and the future value differ by a factor of 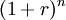$(1+r)^n$; this difference represents the time value of money. It can be concluded that to compare the money available in two different moments it is necessary to consider the time value of money, which allows us to discount money available in the future and consider it in the present or vice versa; only then can we compare the two amounts.

Example 2: Considering an interest rate of 9%, is it better to receive $ 150,000 today or $ 180,000 in three years? What amount of money do you have indifference of choice? If we invest the present value at an interest rate of 9%, in three years we will have a future value of:

$FV = PV * (1 + r)^n = $ 150,000 * (1 + 0.09)^3 = $ 194,254$

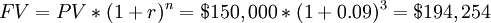
It is better to have $ 150,000 today because by investing it we will have $ 194,254 over three years, which is $ 14,254 more than $ 180,000. To be indifferent, therefore, the amount of money available in three years would have to be $ 194,254.

IRR: definition and formula

To truly understand the essence of the internal rate of return method, a good understanding of the methodological basis of net present value is required as IRR is closely related to it and allows management to add valuable information on decision criteria rather than just look at NPV.

What is the NPV?

Why is useful to know when NPV = 0 (irr)?

$N P V (irr) =\sum_{n=0}^{N} \frac{\left(C F_{n}\right)}{(1+irr)^{n}}$