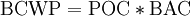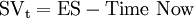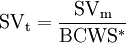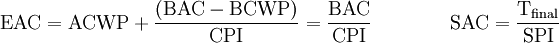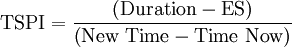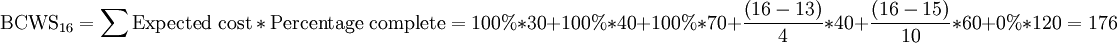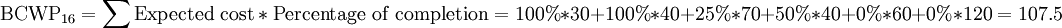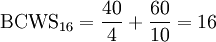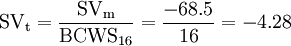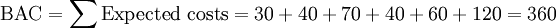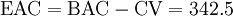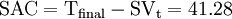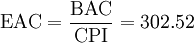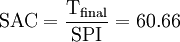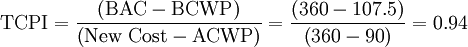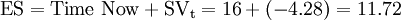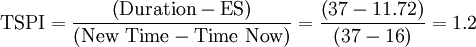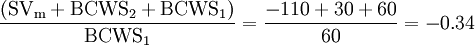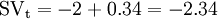Earned value management (EVM)

By Martina Rampazzo

Earned value management (EVM) is a methodology for performance measurement which can be applied to individual projects across programs and portfolios . EVM is used in the planning, executing and controlling phases of the PMI Project Management Standard and mainly involves the knowledge areas of scope, time, cost, communication and integration . More specifically, EVM falls into the project cost management and plan schedule and cost management phases of the PMI Standard of Project Management .

Figure 1: Earned value management for project management (own figure, created based on )

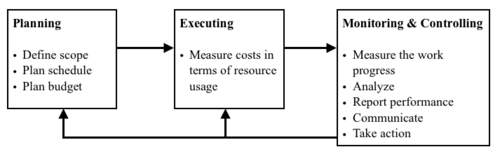
Figure 1 shows how EVM relates to project management phases and the activities which it comprises. While it might appear as mainly related to monitoring and controlling, EVM brings to the project managers’ attention the importance of performance measurement since the planning phase, with the need for accurate planning and for the realization of a project which is easy to execute and measure.

This article will describe the EVM methodology applied to projects, with motivations of its relevance, prerequisites for a successful implementation as well as concrete explanations and applications of each of its key metrics. While EVM is a topic already widely discussed in project management from a theoretical point of view, this article will outline how to apply the tool to practical real life projects. Finally, the main limitations of EVM will be discussed thoroughly, including suggestions for project managers on how to face these challenges.

Why project managers should use EVM?

EVM is a tool created by the U.S. Department of Defense in 1967 and later used to monitor Department of Education projects, such as the U.S. Large Hadron Collider (LHC) of CERN . The ability to connect cost and schedule, to identify project performance indicators and express cost and technical performance in an understandable way, led to the diffusion of this tool and to its increased application in the field of project management . EVM encompasses earned value analysis, which consists in defining a set of indices to monitor the project’s schedule and cost. Subsequently, this data is used to also calculate variance and trend analysis, as well as predictions for the future. Therefore, EVM provides a holistic view on the project’s progress and identifies deviations from the plan and budget, allowing project managers to react to these discrepancies by implementing corrective actions.

The prerequisites for a successful implementation of EVM

There are prerequisites project managers should be aware of in order to implement the methodology in the best possible way. These activities range from planning to monitoring, and consist of:

• Applying the work breakdown structure (WBS - The work breakdown structure in project management) in order to decompose all the projects' activities in control accounts, which are manageable work units with specific scope, cost and schedule. Subsequently, human and material resources have to be allocated to each work package using the organization breakdown structure (OBS) ;
• Assigning management responsibility to each of the control account ;
• Planning how to keep track of physical work progress and how to assign budgetary earned value for each activity ;
• Implementing change management plans to ensure EVM remains relevant if the project scope changes ;
• Using an adequate cost collection system to track actual cost and outstanding invoices ;
• Conducting reporting activities in order to interpret the data obtained and subsequently communicate it to the relevant stakeholders .

How to implement EVM

The EVM methodology comprises four main phases:

1. Defining the time-phased budget
2. Monitoring time and costs
3. Analyzing deviations and defining relevant indices
4. Forecasting

EVM consists in examining the relationship between three types of curves:

• Budgeted Cost of Work Scheduled (BCWS): the planned and scheduled work - also called PV (planned value)

• Budgeted Cost of Work Performed (BCWP): the actual work performed according to the control account - also called EV (earned value)

• Actual Cost of Work Performed (ACWP): the actual expenditures of the control account up to the period of time in which the curve is analyzed - also called AC (actual cost)


Definition of the time-phased budget

The first step of EVM is representing the BCWS, also called the baseline of the project, which represents the cumulative cost of the activities for each period of time and therefore aggregates the budgeted cost of all control accounts. The curve can be obtained thanks to the Gantt chart, which displays the scheduled activities of the project in each unit of time. An example is provided below to clarify this process.

The following set of activities is given, with information on predecessors, scheduled time and budgeted cost.

Activity	Predecessor	Time	Cost
A	/	2	15
B	A	4	20
C	A, B	1	30
D	B	4	10

After creating the Gantt diagram (Gantt Chart in Project Management), the BCWS is drawn so that cumulated costs across all scheduled activities are displayed for each time period. For example, in the first month only activity A occurs, so only its cost (15€) will be added to the BCWS. On the second month, activity A is still taking place so the cumulative cost will be 30€.

• 

  

  Figure 2: Gantt diagram (own figure)
• 

  

  Figure 3: BCWS (own figure)

Monitoring time and costs

Monitoring of costs during the life of a project allows to trace the ACWP, which is the actual cost of work performed. This is extremely relevant as the real costs spent on a project often diverge from the planned ones, as many unforeseen events can occur. Usually actual costs are calculated by checking the actual division of work among manpower and using tools such as cost and time reports, which show how the resources of a project team spent their time and the total amount of expenditures .

Deviation analysis

Figure 4: Possible scenarios of deviation analysis (own figure, created based on )

BCWS and ACWP alone do not provide any information on the nature of a potential deviation. For example, if ACWP > BCWS, it might be that the project either is inefficient, so actual expenditures are higher than the budget, or work is being performed earlier compared to the schedule. For this reason traditional cost management is not precise enough and it is essential to analyze a third curve, the BCWP.

The BCWP can be calculated by multiplying the percentage of completion (POC) with the budget at completion (BAC), which is the total budget of the project. The different methods to calculate the POC will be described further in section 4.

$\mathrm{BCWP}={\mathrm{POC}}*{\mathrm{BAC}}$

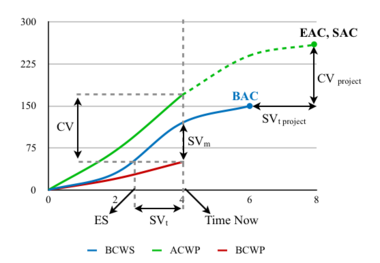
A useful way to conduct deviation analysis is to group the three metrics into one graph, as shown in figure 4. This analysis generates 2ⁿ scenarios, where n is the number of parameters being studied. Being that with EVM the parameters are time and cost, the possible scenarios are four:

1. Project late compared to the schedule and inefficient
2. Project late compared to the schedule and efficient
3. Project on time/early compared to the schedule and efficient
4. Project on time/early compared to the schedule and inefficient

Other than graphically, it is also possible to evaluate time and costs with indices, both relative and absolute, with the former expressing deviations in percentage, while the latter in monetary units.

Relative indices

Relative indices are cost performance index (CPI) and schedule performance index (SPI). If these indices are greater than one, then the project is efficient and ahead of schedule respectively.

$\mathrm{CPI}=\frac{\mathrm{BCWP}}{\mathrm{ACWP}}\qquad\qquad\qquad\qquad \mathrm{SPI}=\frac{\mathrm{BCWP}}{\mathrm{BCWS}}$

Absolute indices

Absolute indices provide the same information of the relative ones but with different formulas, as shown below. In this case, it is preferred to have values larger than 0 for both cost variance (CV) as well as schedule variance (SV_m).

$\mathrm{CV}={\mathrm{BCWP}}-{\mathrm{ACWP}}\qquad\qquad \mathrm{SV_m}={\mathrm{BCWP}}-{\mathrm{BCWS}}$

Figure 5: Earned Schedule (own figure, created based on )

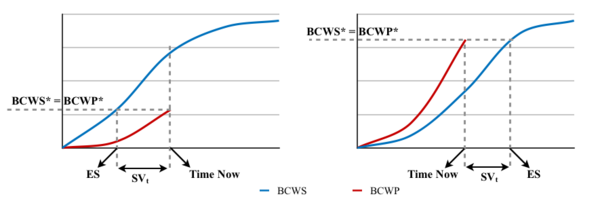
It is important to notice that schedule variance is expressed in monetary units instead of time units, which is more challenging to interpret. This topic was widely discussed from the 1970s, as EVM originally did not include schedule deviations in time units. According to the article Earned Schedule: An Emerging Enhancement to Earned Value Management by Lipke W. and Henderson K., this situation led over time to project managers using the EVM methodology primarily to understand costs rather than schedule . However, in 2002 the earned schedule concept was introduced, a metric which indicates the instant of time in which the BCWS is equal to the BCWP. If these two curves do not match, the projection of the first one onto the second one is the amount of work performed which should be earned according to the schedule (BCWS). The earned schedule is the time from when the project begins up to the projection of this intersection onto the time axis, as shown in figure 5.

In this way, schedule variance can be expressed in time units by subtracting the current period (which in this article will be referred to as Time Now) from the earned schedule. If this value is greater than zero, then it means the project is ahead of schedule.

$\mathrm{SV_t}={\mathrm{ES}}-{\mathrm{Time\ Now}}$

Moreover, if the trend of the BCWS is constant in the interval of time considered, meaning that the same activity is taking place during the entire time, then the schedule variance in time units can also be expressed with the following formula (where BCWS^* is the trend of the curve):

$\mathrm{SV_t}=\frac{\mathrm{SV_m}}{\mathrm{BCWS^*}}$

The case in which the BCWS is not constant will be discussed in section 6 of this article.

Figure 6: Estimate at Completion and Schedule at Completion (own figure, created based on )

Forecasting

By calculating deviation indices, project managers have a picture of the project’s performance in that specific moment. However, before implementing any corrective actions, it is necessary to understand what would be the final arrival point of the project if it continued with the same trend. In fact, identifying, planning, and mobilizing resources to implement corrective actions are time consuming and expensive activities, and may not be necessary if the final performance of the project is within the acceptable range.

The key metrics to forecast future trends are EAC and SAC, which stand for Estimate At Completion and Schedule at Completion. In addition to these metrics, there is also Variance at Completion, which will not be covered in this article (if interested, read more about it at Practice Standard for Earned Value Management of Project Management Institute, p. 19 ). From a graphical point of view, these metrics are obtained by extending the ACWP until the end of the project.

Mathematically, it is important to define which type of effects the deviation analysis in step 3 has on the project. The possible scenarios are three.

Scenario 1: The effect of the issue on the project is limited

This is the case of deviations from original planning due to external factors which impacted the project but have ended their effect. For example, this is the case of higher prices for raw materials and therefore higher costs which impact only limited initial activities of the project.

$\mathrm{EAC}={\mathrm{BAC}}-{\mathrm{CV}}\qquad\qquad\qquad\qquad\qquad\qquad \mathrm{SAC}={\mathrm{T_{final}}}-{\mathrm{SV_t}}$

Scenario 2: The effect of the issue on the project is extended

The second scenario corresponds to an issue impacting not only current activities, but also the entire project. There are also other alternatives to this calculation which can be found at Practice Standard for Earned Value Management of Project Management Institute, pg. 21 .

$\mathrm{EAC}={\mathrm{ACWP}}+\frac{\left ({\mathrm{BAC}}-{\mathrm{BCWP}} \right )}{\mathrm{CPI}}=\frac{\mathrm{BAC}}{\mathrm{CPI}}\qquad\qquad \mathrm{SAC}=\frac{\mathrm{T_{final}}}{\mathrm{SPI}}$

Scenario 3: Both types of issues affect the project

Figure 7: Forecasting steps for Scenario 3 (own figure, created based on )

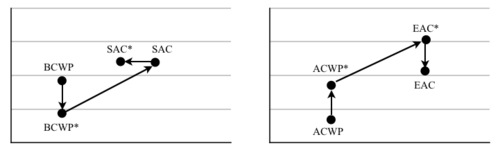
Practically speaking, it is possible that the project falls into a grey zone in between the two scenarios and that there are two components of a deviation, one for each of the two scenarios. In this case, studies from the Department of Management, Economics and Industrial Engineering of Politecnico di Milano suggest the following steps to calculate EAC and SAC . The suggested process is valid if cost and time deviations of scenario 1 are efficiency (Lower cost₁ in the formula) and anticipation compared to the schedule (Earlier schedule₁ in the formula). In the opposite case in which scenario 1 deviations are inefficiency and lateness, the formulas of step 1 and 3 will have the opposite sign.

• Remove the positive deviations with limited effect and calculate the new ACWP* and BCWP*;

$\mathrm{ACWP^*}={\mathrm{ACWP}}+{\mathrm{Lower\ cost_1}}\qquad\qquad \mathrm{BCWP^*}={\mathrm{BCWP}}-{\mathrm{Earlier\ schedule_1}}$

• Calculate CPI* and SPI* and the corresponding EAC* and SAC* with the values obtained in step 1. These are the forecasted values which account only for deviations of scenario 2;
• Calculate EAC and SAC by reintroducing the deviations of scenario 1

$\mathrm{EAC}={\mathrm{EAC^*}}-{\mathrm{Lower\ cost_1}}\qquad\qquad \mathrm{SAC}={\mathrm{SAC^*}}-{\mathrm{Earlier\ schedule_1}}$

To Complete Indices

The last step of EVM consists in defining the SPI and CPI indices the project should have in order to reach the desired schedule and cost targets. To evaluate their actual concrete feasibility, To Complete Performance Index (TCPI) and To Complete Schedule Performance Index (TSPI) are calculated:

$\mathrm{TCPI}=\frac{ {\left ({\mathrm{BAC}}-{\mathrm{BCWP}} \right )}} {\left ({\mathrm{New\ Cost}}-{\mathrm{ACWP}} \right )}$

$\mathrm{TSPI}=\frac{ {\left ({\mathrm{Duration}}-{\mathrm{ES}} \right )}} {\left ({\mathrm{New\ Time}}-{\mathrm{Time\ Now}} \right )}$

Project managers should interpret these indices to understand whether it is really feasible to maintain them until the end of the project. A research conducted by the U.S. Department of Defense concluded that once a work breakdown element (WBE) is over 20% of its completion, CPI and SPI indices should not be increased by 10% because the WBE is considered stable and therefore the final performance of a project will not diverge significantly.

Percentage of Completion Calculation Methods (POC)

As explained previously, in order to calculate the BCWP, project managers need to estimate the POC, which describes the actual progress of the project. There are many methods to evaluate progress, some objective and others subjective. This article will divide the POC calculation methods according to the division presented in the article Analysis and application of earned value management to the Naval Construction Force, pp.18-20 and will briefly explain the most relevant limitations.

1. Discrete effort:

   • Milestone Method: assigns a weight to each milestone. Once this is completed, the percentage of completion will increase of the value of the milestone. It suits longer projects and requires more than 2 milestones;
   • Fixed Ratio Formula Method: a variation of the previous method which assumes only 2 milestones, one at the beginning of an activity and the second one at the end, with weights that may vary but have to add up to 100%. The most common ratios used are 0/100, 50/50 and 25/75;
   • Equivalent Unit Method: consists in counting the number of completed units and comparing it to the total units which need to be accomplished. It is applicable only if the activities allow to count physical units, therefore it suits repetitive tasks;
   • Percent Complete Method: applies a percentage of completion according to the progress of the activity. It is easier to apply for projects where performance is measurable. It is also used as a subjective method where the control account manager estimates the percentage of completion according to his experience and knowledge;
   • Percent Complete with Milestone Gates Method: a variation of the milestone method which allows to report subjective percentages of completion to the activity until the milestone is reached;
   • Earned Standards: determines the percentage of completion according to standards derived from historical data, time and motion studies and other methods. It is challenging to apply one unique standard as every project has its own specific characteristics and is influenced by factors which vary from project to project.

2. Apportioned Effort: associates activities which are closely correlated in terms of planning and performance. An example is using the same percentage of completion for both production control and production.
3. Level of Effort (LoE): it assumes that the progress of activities is proportional to time. This method should be avoided as it assumes that BCWP is always the same as BCWS. In this way, SV and SPI will always be equal to 0 and 1 respectively and misleading cost variances will be generated .

As a result, some methods are by nature more subjective, like level of effort, apportioned effort, percent complete method, while others are more objective and therefore suit controlling activities, such as milestone, fixed formula and equivalent unit methods. Project managers should avoid the use of subjective methods since their level of accuracy is questionable and, if not completely possible, impose a limit on the number of activities controlled with such logics .

Application of EVM

The following section provides a practical application of the concepts of EVM explained in the previous chapters. The assumption of this example is that, at the beginning of day 16, controlling and monitoring activities are conducted to evaluate the performance of the project. The EVM structure previously discussed will be applied in this example step by step.

Definition of the time-phased budget

The following table is given, with activities, predecessors, planned duration and costs.

Activity	Predecessor	Expected Duration	Expected Cost
1	/	5	30
2	1	8	40
3	1	10	70
4	2	4	40
5	3	10	60
6	4, 5	12	120

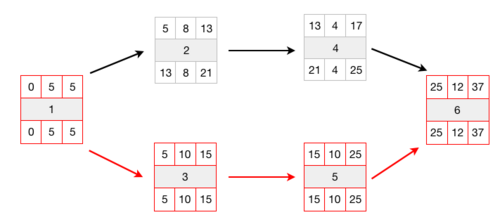
To calculate the BCWS, it is useful to create the network of the project in order to check which activities should have been completed on day 16 (figure 8, where the critical path has been highlighted in red).

Figure 8: Network and critical path (own figure)

$\mathrm{BCWS_{16}}=\mathrm{\sum Expected\ cost*Percentage\ complete}={\mathrm{100%}}*{\mathrm{30}}+{\mathrm{100%}}*{\mathrm{40}}+{\mathrm{100%}}*{\mathrm{70}}+\frac{ {\left ({\mathrm{16}}-{\mathrm{13}} \right )}}{\mathrm{4}}*{\mathrm{40}}+\frac{ {\left ({\mathrm{16}}-{\mathrm{15}} \right )}}{\mathrm{10}}*{\mathrm{60}}+{\mathrm{0%}}*{\mathrm{120}}={\mathrm{176}}$

Monitoring time and costs

It is assumed that the monitoring of costs results in ACWP₁₆=90.

Deviation analysis

In this example, the percentage of completion method is used to calculate BCWP and it is assumed that the control account manager provided the following information:

Activity	Percentage of Completion
1	100%
2	100%
3	25%
4	50%
5	0%
6	0%

Therefore the BCWP in day 16 is given by:

$\mathrm{BCWP_{16}}=\mathrm{\sum Expected\ cost*Percentage\ of\ completion}={\mathrm{100%}}*{\mathrm{30}}+{\mathrm{100%}}*{\mathrm{40}}+{\mathrm{25%}}*{\mathrm{70}}+{\mathrm{50%}}*{\mathrm{40}}+{\mathrm{0%}}*{\mathrm{60}}+{\mathrm{0%}}*{\mathrm{120}}={\mathrm{107.5}}$

Relative indices

$\mathrm{CPI}=\frac{\mathrm{BCWP}}{\mathrm{ACWP}}={\mathrm{1.19}}\qquad\qquad\qquad\qquad \mathrm{SPI}=\frac{\mathrm{BCWP}}{\mathrm{BCWS}}={\mathrm{0.61}}$

Absolute indices

$\mathrm{CV}={\mathrm{BCWP}}-{\mathrm{ACWP}}={\mathrm{17.5}}\qquad\qquad \mathrm{SV_m}={\mathrm{BCWP}}-{\mathrm{BCWS}}={\mathrm{-68.5}}$

The indices show that the project is efficient and late compared to the schedule.

It is also useful to calculate the schedule variance expressed in time units to verify how much days the project is late. In this exercise, the trend of the BCWS in day 16 is constant as no new activity is started and no activity is finished compared to day 15. In this time period only activities 4 and 5 are occurring, therefore only their expected costs and durations are considered:

$\mathrm{BCWS_{16}}=\frac{\mathrm{40}}{\mathrm{4}}+\frac{\mathrm{60}}{\mathrm{10}}={\mathrm{16}}$

$\mathrm{SV_{t}}=\frac{\mathrm{SV_m}}{\mathrm{BCWS_{16}}}=\frac{\mathrm{-68.5}}{\mathrm{16}}={\mathrm{-4.28}}$

Forecasting

In this example, scenarios 1 and 2 for forecasting are applied. The calculations show that issues with an impact on the entire project tend to amplify the effect on the esteems both in case of positive and negative deviations.

Scenario 1: The effect of the issue on the project is limited

$\mathrm{BAC}=\mathrm{\sum Expected\ costs}= {\mathrm{30}} + {\mathrm{40}}+{\mathrm{70}}+{\mathrm{40}}+{\mathrm{60}}+{\mathrm{120}}={\mathrm{360}}$

$\mathrm{EAC}={\mathrm{BAC}}-{\mathrm{CV}}={\mathrm{342.5}}$

$\mathrm{SAC}={\mathrm{T_{final}}}-{\mathrm{SV_t}}={\mathrm{41.28}}$

Scenario 2: The effect of the issue on the project is extended

$\mathrm{EAC}=\frac{\mathrm{BAC}}{\mathrm{CPI}}=\mathrm{302.52}$

$\mathrm{SAC}=\frac{\mathrm{T_{final}}}{\mathrm{SPI}}=\mathrm{60.66}$

To Complete Indices

$\mathrm{TCPI}=\frac{ {\left ({\mathrm{BAC}}-{\mathrm{BCWP}} \right )}} {\left ({\mathrm{New\ Cost}}-{\mathrm{ACWP}} \right )}=\frac{ {\left ({\mathrm{360}}-{\mathrm{107.5}} \right )}} {\left ({\mathrm{360}}-{\mathrm{90}} \right )}={\mathrm{0.94}}$

$\mathrm{ES}={\mathrm{Time\ Now}}+{\mathrm{SV_t}}={\mathrm{16}}+{\mathrm{(-4.28)}}={\mathrm{11.72}}$

$\mathrm{TSPI}=\frac{ {\left ({\mathrm{Duration}}-{\mathrm{ES}} \right )}} {\left ({\mathrm{New\ Time}}-{\mathrm{Time\ Now}} \right )}=\frac{ {\left ({\mathrm{37}}-{\mathrm{11.72}} \right )}} {\left ({\mathrm{37}}-{\mathrm{16}} \right )}={\mathrm{1.2}}$

Coherently with the results in step 3, the to complete indices suggest that CPI index can be worsened, while to be able to finish the project in day 37, SPI has to be improved from 0.61 to 1.2, which corresponds to a 96.72% increase. According to criteria of the U.S. Department of Defense explained in section 3.5, this is unlikely to occur.

Practical real life case for schedule variance calculation

Figure 9: Example of practical case for Schedule Variance calculation (own figure, created based on )

During the monitoring of projects, often real life situations are not as expected from a theoretical point of view. In section 3.3 schedule variance expressed in time units was discussed, under the circumstances that BCWS curve does not change trend in the considered period of time. The formula to calculate it is:

$\mathrm{SV_t}=\frac{\mathrm{SV_m}}{\mathrm{BCWS^*}}$

However, there are some situations in which this formula is not accurate and may approximate too much the value of SV_t. According to studies from the Department of Management, Economics and Industrial Engineering of Politecnico di Milano , a way to verify this is to analyze the BCWS in the periods of time which range from the Earned Schedule to Time Now. If its trend is constant, then the simplified formula can be applied. Otherwise, the following pragmatic steps are suggested:

1. Subtract (or add if SV_m is negative) from SV_m the values of BCWS_period for each time period until SV_m is equal to 0 (which corresponds to the point in which BCWS and BCWP meet). This will return the interval to which SV_t belongs to;
2. To determine the decimal values, use the simplified formula. The numerator corresponds to the remaining SV_m after having subtracted BCWS_period in step 1, while the denominator is the BCWS_period in the period of time of SV_t.

An example is presented to better understand this process.

In figure 9 it can be seen that BCWS changes trend from ES to Time Now. Under the hypothesis that SV_m = -110€, BCWS₁ = 60 and BCWS₂ = 30, applying the simplified formula of section 3.3 returns SV_t = -3.67. However, looking at the graph it can be seen that it is actually in between -2 and -3. Applying step 1, SV_m is closest to 0 when adding BCWS₂ and BCWS₁. This corresponds to SV_t being smaller than -2. Finally, applying step 2, the decimals are:

$\frac{\left ({\mathrm{SV_m}}+{\mathrm{BCWS_2}}+{\mathrm{BCWS_1}} \right )}{\mathrm{BCWS_1}}=\frac{\mathrm{-110+30+60}}{\mathrm{60}}=\mathrm{-0.34}$

Therefore:

$\mathrm{SV_t}=\mathrm{-2}+\mathrm{0.34}=\mathrm{-2.34}$

This approach is effective as it provides an accurate value for SV_t when the trend of the BCWS is not constant, however it may be challenging to implement for large projects where changes in the trend of the BCWS occur often.

Limitations

The following section includes limitations of EVM, as discussed in a variety of articles and books.

• First of all, the calculation of the POC and thus the BCWP curve is a challenging part of EVM as there is a wide range of methods which can be used, as discussed in section 4 of this article. Project managers have to evaluate the trade-off between the inaccuracy of subjective methods and the demanding calculations required by discrete methods. Moreover, the choice of the tool for the BCWP calculation is not a controlling but a planning activity and therefore, when planning each activity, it is important to define also what is the best way to control it . Another aspect is that being that POC and BCWP are often approximated values, all the following indices calculated may be imprecise and thus should always be interpreted according to the context of the project.

• Another problematic aspect concerns the application of formulas for WBEs at the initial stages of completion. Looking at EAC formula in section 3.4, the less work is done, the more BCWP will vary from BAC and EAC will diverge from ACWP, the actual work performed. This suggests that forecasting should be carried out with caution especially in the initial phases of the project, as small variations in SPI and CPI indices from 1, can lead to huge variations in the time and cost of the activities, which often do not correspond to reality. An example from the book L'organizzazione dell'impresa by Bartezzaghi Emilio will be taken into consideration. The first assumption is that there are two WBEs, one which is in the early stage (lower than 20% of completion) and another one in the final phases. The second assumption is that these two activities are on time and have the same negative cost variance. According to the formulas, CPI₁ is smaller than CPI₂ because WBE₁ has just begun. According to the same logic, EAC₁ is greater than EAC₂ (scenario 2 of forecasting). This suggests that WBE₂ is performing better than WBE₁. However, when calculating the TCPI, WBE₂ should increase its performance tremendously compared to WBE₁ in order to finish the project with the planned total budget. This is due to the fact that WBE₂ is nearly finished and therefore it has less time to make up for the cost deviations. For this reason, project managers should impose a limit of variation of esteems of +/- 10% if the activity is already stable, so usually for BCWP=20%*BAC .

• Another limitation to EVM with regards to forecasting is that there is always some degree of uncertainty on the different parameters that characterize a project. These parameters include the “activity durations or costs, resource use, the presence of precedence relations or even on the existence of an activity in the project network” (Vanhoucke M., 2016, Integrated Project Management Sourcebook, p. 237). This makes forecasting in EVM a critical step that project managers have to approach carefully. A suggested solution proposed by Vanhoucke M. is for project managers to visualize the future trend of these parameters not as a point, but as a dataset, and therefore as a probability distribution. In this way, it is possible to apply simulation models that imitate the progress of parameters and to understand the overall future trend of the project. More on this can be read in the article RA4: Activity distributions, p. 119 .

• Another critical point of EVM project managers should be aware of is in case of collaborations with external suppliers. If contracts are based on fixed prices, suppliers are not obliged to reveal their structure of costs. Hence, if there are issues with activities based on supply, it is possible to detect possible lateness but not inefficiency. This missing transparency on costs leads to CPI always being 1 and therefore to a lower level of accuracy during the controlling phase .

• Finally, EVM focuses only on schedule and costs of a project but lacks information on risk and quality, which are key aspects to determine the success of a project. The article Project Management Model: Integrating Earned Schedule, Quality, and Risk in Earned Value Management suggests new indices to combine with traditional EVM in order to measure quality performance and risk assessment .

Annotated Bibliography

This section includes references for further reading on EVM and its application to project management:

• Project Management Institute, Inc. (2005). Practice Standard for Earned Value Management. Pennsylvania, USA: Project Management Institute, Inc.: this book represents the standards for EVM methodology, with a strong focus on how it is connected to the project management process and when project managers should apply it. It provides a detailed list of the steps of the methodology as well as considerations on how EVM fits different situations depending on the degree of uncertainty and significance of the project as well as the frequency and granularity of the performance measurement. The final chapters are then dedicated to the prerequisites for the application of EVM and guidance on the use of its key metrics.
• Hayes, R. (2001). Analysis and application of earned value management to the Naval Construction Force. California, USA: Naval Postgraduate School (U.S.). Dudley Knox Library: this paper provides a concise description of EVM and summarizes the several findings of published literature on this topic. The first part of the paper also provides information on the origin of the methodology within government and how it evolved throughout the years to become a more user-friendly tool applicable also to private industries. Finally, it provides a practical application to Naval Construction Force project management, not only as a mere calculation of the indices but also as an analysis of the planning and execution phases, such as project monitoring and reporting.
• Vanhoucke, M. (2016). Integrated Project Management Sourcebook. Gent, Belgium: Springer: this book deals with the theme of Integrated Project Management and Control, tackling not only EVM methodology, but also network and resource analysis, scheduling techniques such as PERT and Critical Path Method, schedule risk analysis, buffer management and more. In fact, it comprises of +70 articles which discuss about topics directly linked to EVM. In this way, it allows the reader to have a more holistic view and knowledge of baseline scheduling, risk analysis and project control and therefore understand how to combine EVM with other tools and techniques for project planning, execution and controlling.
• Miguel, A., Madria, W. and Polancos, R. (2019). Project Management Model: Integrating Earned Schedule, Quality, and Risk in Earned Value Management. In: 2019 IEEE 6th International Conference on Industrial Engineering and Applications. De La Salle University Manila, Philippines: Department of Industrial Engineering: this article provides project managers with an updated version of EVM which allows to overcome one of the most important limitations of this tool, which is the lack of quality and risk performance measurement. A new model is proposed and demonstrates how quality problems affect the cost and schedule of a project and how, from risk indices, preventive actions can be taken. Moreover, these new indices are applied to a sample project in order to validate it from a practical point of view. Finally, this article highlights how the new model allows to identify more accurate schedule deviations and thus represent the real time delay.