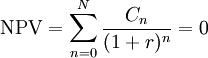Internal Rate of Return (IRR)

Developed by Pablo Leandro Capellari- s213666

Internal rate of return (IRR) is a parameter used in the financial analysis to determine the profitability of the investment, that is, it estimates the rate of return that the evaluated project could have. The term "internal" is because for the calculation of the IRR, external factors that could affect the profitability of the project, such as inflation, are not considered. In mathematical terms, the IRR is defined as the discount rate that causes the sum of the cash flows of the project to be zero. In other words, if the net present value (NPV) of a project is 0 at a certain rate, that rate is the IRR. Researchers in 2003 have validated the IRR as an alternative to the NPV as an indicator for project evaluation, considering that the IRR as from the point of view of the investor and the NPV from the point of view of the society. Its popular application is mainly because it defines the return on investment, sometimes seen as a measure of efficiency, beyond its documented limitations. For decision-makers, the IRR can be compared to the discount rate for rejecting or accepting projects. To be specific, for a project to be accepted, the IRR must be greater than the discount rate and when both are equivalent, a situation of indifference arises. To resolve many deficiencies related to the traditional IRR, some researchers have proposed to use the average internal rate of return (AIRR). The AIRR is based on the breakdown of the NPV of the project between the invested capital and the economic efficiency, maintaining the consistency of the NPV to decide the acceptance of a project. This breakdown generates valuable information helping to determine the uncertainty of a project allowing risk to be explored in more detail.

Big idea

Describe the tool, concept or theory and explain its purpose. The section should reflect the current state of the art on the topic

IRR Mathematically

IRR is the discount rate (interest rate) that makes the sum of the present value of the cash flows zero. If the NPV of the project is zero at the selected discount rate, then that discount rate is, by definition, the IRR. IRR is algebraically equivalent. Mathematically expressed as:

$\operatorname{NPV} = \sum_{n=0}^N \frac{C_n}{(1+r)^n} = 0$

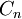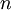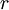where $C_n$ is a cash flow in period $n$ and $r$ is the IRR.

Practical example

In the following example, a highway construction project is outlined to demonstrate the calculation of IRR and other parameters. For exemplification purposes, the following data have been used: The project opens in year 0 and has an evaluation period on 10 years, the project has the following first year impacts: –Time savings: 500,000 DKK. –Accident savings: 50,000 DKK. Operation and maintenance: 100,000 DKK.

Assumptions

Discount rate	0,03
Traffic growth	0,015
Price growth	0,01

Assumptions

Year	-	-3	-2	-1	0	1	2	3	4	5	6	7	8	9	10
Discount factor	-	1,09	1,06	1,03	1,00	0,97	0,94	0,92	0,89	0,86	0,84	0,81	0,79	0,77	0,74
Traffic growth	-	0	0	0	1,00	1,02	1,03	1,05	1,06	1,08	1,09	1,11	1,13	1,14	1,16
Price growth	-	0	0	0	1,00	1,01	1,02	1,03	1,04	1,05	1,06	1,07	1,08	1,09	1,10
Construction costs	,-10.612.090	-3.642.423	-3.536.333	-3.433.333
Scrap value	7.440.939
Operation and maintenance	-953.020	-	-	-	-100.000	-97.087	-94.260	-91.514	-88.849	-86.261	-83.748	-81.309	-78.941	-76.642	-74.409
Time savings	5.372.322	-	-	-	500.000	497.646	495.302	492.970	490.649	488.338	486.039	483.750	481.473	479.205	476.949
Accidents	476.510	-	-	-	50.000	48.544	47.130	45.757	44.424	43.130	41.874	40.655	39.470	38.321	37.205
NPV	1.724.661
BCR	1,16
IRR	4,57%

Application

Provide guidance on how to use the tool, concept or theory and when it is applicable

Limitations

Critically reflect on the tool/concept/theory. When possible, substantiate your claims with literature