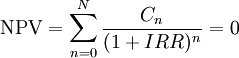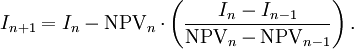Internal Rate of Return (IRR)
Developed by Pablo Leandro Capellari- s213666
Internal rate of return (IRR) is a parameter used in the financial analysis to determine the profitability of the investment, that is, it estimates the rate of return that the evaluated project could have. The term "internal" is because for the calculation of the IRR, external factors that could affect the profitability of the project, such as inflation, are not considered. In mathematical terms, the IRR is defined as the discount rate that causes the sum of the cash flows of the project to be zero[1]. In other words, if the Net Present Value (NPV) of a project is 0 at a certain rate, that rate is the IRR[2]. Researchers in 2003[3] have validated the IRR as an alternative to the NPV as an indicator for project evaluation, considering that the IRR as from the point of view of the investor and the NPV from the point of view of the society. Its popular application is mainly because it defines the return on investment, sometimes seen as a measure of efficiency, beyond its documented limitations. For decision-makers, the IRR can be compared to the discount rate for rejecting or accepting projects. To be specific, for a project to be accepted, the IRR must be greater than the discount rate and when both are equivalent, a situation of indifference arises[1]. To resolve many deficiencies related to the traditional IRR, some researchers[4] have proposed to use the average internal rate of return (AIRR). The AIRR is based on the breakdown of the NPV of the project between the invested capital and the economic efficiency, maintaining the consistency of the NPV to decide the acceptance of a project. This breakdown generates valuable information helping to determine the uncertainty of a project allowing risk to be explored in more detail.
Contents |
BIG IDEA
It is possible to consider a successful investment as one that manages to reach or exceed the expected returns, and investors tend to analyze how this type of investment has behaved in the past to have a vision of what could happen in the future. It is clear that past performance is not a guarantee of what might happen in the future, but it is an important influence on financial attractiveness. If the cash flow turns out to be as predicted, that the asset was valued rationally, the investor gets the expected rate of return. On the other hand, if the return is higher than expected, the actual return will be higher than the target rate.Therefore, different types of rates can be defined: a) the required rate of return: which is the target rate measured at the beginning of the investment, b) the real rate of return: which is the rate measured at the end of the project. In the market, both rates are known as IRR.[2]
General usage of IRR
As mentioned earlier, the IRR is a investment decision-making tool used to determine the viability of various investment options. The general criteria for understanding suitability for a project are as follows:
- if TIR > r
 project is accepted. The reason for this is that the project generates a higher rate of return than the required minimum rate of return (opportunity cost), which means we will make more money than investing in a specific project (IRR), for example, buying government bonds.
project is accepted. The reason for this is that the project generates a higher rate of return than the required minimum rate of return (opportunity cost), which means we will make more money than investing in a specific project (IRR), for example, buying government bonds.
- If TIR < r
 project will be rejected. The reason is that the project's return is lower than the required minimum return. This shows that if we invest in government bonds (r), we will make more money than if we invest in specific projects (IRR).
project will be rejected. The reason is that the project's return is lower than the required minimum return. This shows that if we invest in government bonds (r), we will make more money than if we invest in specific projects (IRR).
- If TIR = r
 it would be a situation of indifference, similar to the one that occurs when the NPV is equal to zero.
it would be a situation of indifference, similar to the one that occurs when the NPV is equal to zero.
r= discount rate
IRR Mathematically[2]
IRR is the discount rate (interest rate) that makes the sum of the present value of the cash flows zero. If the NPV of the project is zero at the selected discount rate, then that discount rate is, by definition, the IRR. IRR is algebraically equivalent. Mathematically expressed as:
where 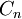 is a cash flow in period
is a cash flow in period 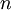
Numerical solution
Another alternative to calculate the  is the secant method:
is the secant method:
where  is considered the
is considered the 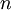 th approximation of the IRR.
th approximation of the IRR.
Different levels of precision can be used to calculate  , for example Microsoft Excel and Google Sheets have default functions for the calculation; "=IRR(...)" and "=XIRR(...)"
, for example Microsoft Excel and Google Sheets have default functions for the calculation; "=IRR(...)" and "=XIRR(...)"
The convergence behaviour of by the following:
- If the function
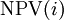 has a single real number root
has a single real number root  , then the sequence converges reproducibly towards
, then the sequence converges reproducibly towards 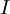 .
.
- If the function
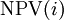 has
has 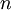 real roots
real roots 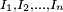 , then the sequence converges to one of the roots, and changing the values of the initial pairs may change the root to which it converges.
, then the sequence converges to one of the roots, and changing the values of the initial pairs may change the root to which it converges.
- If function
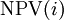 has no real roots, then the sequence tends towards +∞.
has no real roots, then the sequence tends towards +∞.
Having 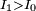 when
when 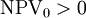 or
or 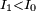 when
when 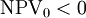 may speed up convergence of
may speed up convergence of  to
to 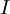 .
.
Practical example
In the following example, a highway construction project is outlined to demonstrate the calculation of IRR and other parameters. For exemplification purposes, the following data have been used: The project opens in year 0 and has an evaluation period on 10 years, the project has the following first year impacts: –Time savings: 500,000 DKK. –Accident savings: 50,000 DKK. Operation and maintenance: 100,000 DKK.
| Discount rate | 0,03 |
|---|---|
| Traffic growth | 0,015 |
| Price growth | 0,01 |
In addition, different reference values have been used for this type of project, such as a discount rate of 3%, annual traffic growth of 3% and price growth of 1%.
| Year | - | -3 | -2 | -1 | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Discount factor | - | 1,09 | 1,06 | 1,03 | 1,00 | 0,97 | 0,94 | 0,92 | 0,89 | 0,86 | 0,84 | 0,81 | 0,79 | 0,77 | 0,74 |
| Traffic growth | - | 0 | 0 | 0 | 1,00 | 1,02 | 1,03 | 1,05 | 1,06 | 1,08 | 1,09 | 1,11 | 1,13 | 1,14 | 1,16 |
| Price growth | - | 0 | 0 | 0 | 1,00 | 1,01 | 1,02 | 1,03 | 1,04 | 1,05 | 1,06 | 1,07 | 1,08 | 1,09 | 1,10 |
| Construction costs | ,-10.612.090 | -3.642.423 | -3.536.333 | -3.433.333 | |||||||||||
| Scrap value | 7.440.939 | ||||||||||||||
| Operation and maintenance | -953.020 | - | - | - | -100.000 | -97.087 | -94.260 | -91.514 | -88.849 | -86.261 | -83.748 | -81.309 | -78.941 | -76.642 | -74.409 |
| Time savings | 5.372.322 | - | - | - | 500.000 | 497.646 | 495.302 | 492.970 | 490.649 | 488.338 | 486.039 | 483.750 | 481.473 | 479.205 | 476.949 |
| Accidents | 476.510 | - | - | - | 50.000 | 48.544 | 47.130 | 45.757 | 44.424 | 43.130 | 41.874 | 40.655 | 39.470 | 38.321 | 37.205 |
| NPV | 1.724.661 | ||||||||||||||
| BCR | 1,16 | ||||||||||||||
| IRR | 4,57% | ||||||||||||||
| (Simple example of the calculation of the IRR. Developed by Pablo Capellari s213666. | |||||||||||||||
The table displays year-over-year cash flows, plus calculations for Residual Value, Net Present Value (NPV), Benefit Cost Rate (BCR), and Internal Rate of Return (IRR). For the calculation of the IRR, the Excel function "Goal Seek" has been used, where it allows estimating the IRR generating an NPV=0. Another option that can be used to calculate the IRR is the =IRR(cash flows) function in Excel.
Negative IRRs
In simple terms, a negative IRR indicates that the cash flows of a project are not enough to cover the initial investment. Therefore, this means that the project is not attractive to the investor because he would lose money. In such situations, the NPV would also be negative. On the other hand, a negative NPV value does not always mean a negative IRR, it just means that the discount rate is greater than the IRR. The following simple example allows us to clarify the concept of negative IRR.
| Year | Year 0 | Year 1 | Year 2 | Year 3 | Year 4 |
|---|---|---|---|---|---|
| Cash Flow | .-300.000,00 kr. | 30.000,00 kr. | 35.000,00 kr. | 50.000,00 kr. | 80.000,00 kr. |
| IRR | .-13% | ||||
| (Simple example of the calculation of a negative IRR. Developed by Pablo Capellari s213666. | |||||
The sum of the cash flows is negative, so it generates a negative IRR, in this case the initial investment is 300.000,00 kr (Danish kroner) and the income in the four-year period adds up to a total of 195.000,00 kr (Danish kroner), for so the IRR is -13%.
APPLICATION
IRR for project managers
Risk Assessment in portfolios, programs and projects has the objective of defining the action patterns of an organization respecting its vision and values. Therefore, in recent years, risk analysis for the proper management of portfolios and projects has been evolving according to the dynamics and needs of the market, as well as the company's internal strategies. The vast majority of project risk assessments are limited to Cost-Benefit Analysis (CBA), numerically determining economic data (NPV, BCR, IRR). It is important to note that when evaluating a project it is essential to consider social and environmental criteria (economic development, public image, environmental sustainability), for example with the use of Multi-Criteria Decision Analysis (MCDA).[5]
The purpose of Cost-Benefit Analysis (CBA) is to compare different projects considering both financial flows and economic impacts on society, in a common and objective monetary metric. CBA can be used in projects of all scales, from large projects such as public infrastructure to smaller projects of a private nature.Public policy has many goals, affects different groups of beneficiaries, and spans different time horizons. The advantage of the cost-benefit analysis method is to simplify the multidimensionality of the problem by calculating the monetary value of each key benefit and cost. To make these elements fully comparable, the method converts all economic and financial flows observed at different times into current values. This method, called discounting, is essential for cost-benefit analysis. This allows projects to be evaluated according to how much society values the well-being of future generations. The idea is that benefits or costs are more valuable when they have a direct impact. Consequently, activities that result in high costs or benefits to future generations appear to be less important. The key here is to weight the time period with an appropriate discount factor.[6]
The CBA can be elaborated under different methodologies, as well as the great variety of results that it is possible to obtain from it. The most relevant investment criteria are NPV, IRR and BCR. Net present value (NPV) considers the difference between benefits (impacts users and other benefits) and costs (investment and maintenance costs), discounted over the entire duration of the project. The criterion used to determine the profitability of the project is NPV>0, and when comparing projects, the project with the highest NPV should be chosen according to this criterion. It is clear that there are many factors involved in this decision, so the NPV should not be the only factor to consider. Therefore, the IRR also participates, since it determines the discount rate which balances costs and benefits of the project, which serves as a complement to the NPV criterion in the CBA. This being the main reason why the IRR is a tool for project managers at the time of project evaluation. Another important criterion in the CBA is the Benefit-Cost Rate (BCR), which considers the ratio between the discounted benefits and the discounted costs throughout the duration of the project. The use of the BCA is recommended for budget-constrained projects, which are also independent between each other. And the selection criteria for a profitable project is to have BCR>1. [5]
IRR in real-estate investment
It is common opinion that the Internal Rate of Return (IRR) may be the real estate industry's preferred performance measure as a means of evaluating the return on projected investments. However, finance textbooks[7] pointed out that the net present value (NPV) is a superior method to the IRR used to evaluate potential investments, however, in practice, the internal rate of return return still dominates. This is an easy-to-understand indicator that the appeal is that it meets the need for a single number compared to other opportunities or benchmarks. This simplicity belies its true nature and many of the problems that can arise when it is used to evaluate capital investment projects.[2]
IRR limitations on real state investments
- Does not express a measure of the size or timing of the project.
- Does not differentiate between sales revenues or cash flows from operations.
- Does not demonstrate the timing of cash flows.
- Assume cash flows can be reinvested.
- Does not differentiate risk levels between years within the project.
- No express leverage risk.
- Not influenced by real estate, lease, operating or liquidity risk.
IRR vs NPV for project ranking
In situations where it is necessary to choose between mutually exclusive projects, it can usually happen that there are differences in the results of the analyzes between the NPV and the IRR, which often causes problems for decision-makers. The use of the IRR is disputed for various reasons, but it is still widely used in practice [8]. The IRR tends to express the return on invested capital, a project should be accepted if the IRR is greater than the interest rate, because this case would generate a positive NPV [9]. This means that for these situations both indicators are consistent and the acceptance of a single project would be facilitated. For mutually exclusive projects, where these indicators rank in a different order than the alternatives, it is usually explained by the "investment assumption", which establishes that the differences between intermediate cash flows must be recognized. For these situations, the investor must consider what will happen to the differences in cash flows in between. For example, if project A has an initial investment of 200,000 kr (Danish kroner) and another project B an initial investment of 150,000 kr (Danish kroner), the decision-maker must consider what happens with that difference of 50,000 kr (Danish kroner). It is assumed that in this example, the NPV calculation is higher in project B, while the IRR is higher in project A. Therefore, the investor will have uncertainty between both projects, depending on whether to rely on the criterion of NPV or IRR. This author [8]proposes the use of the Investment Difference (DI), by which it is intended to compare the cash flows of mutually exclusive projects.
LIMITATIONS
The IRR is generally used by decision makers to choose which of the investment alternatives is the most attractive, unfortunately this rate has several defects:
Mutually Exclusive Projects
This limitation is related to the "IRR vs NPV for project ranking" section because if it is the only indicator used, this could cause erroneous decisions when choosing a project. This type of problem arises when the projects have different values of initial investment, let's suppose project A with an initial investment of 10,000 kr, an NPV of 80,000 kr, and an IRR of 100%. On the other hand, a project B with an initial investment of 100,000 kr, an NPV of 360,000 kr, and an IRR of 50%. Considering the IRR criterion alone, the investor could decide on project A (IRR=100%), but project B (IRR=50%) would generate 280,000 kr more. It is to be expected that in a project with higher investment, the profits are also higher. The analysis would be to invest 10 times in project A with the same investment used for project B, of course, if this were feasible. From another point of view, focusing the analysis on the NPV over the IRR would generate discarding the most profitable in percentage terms. A practical alternative to solve this situation from different perspectives is normalizing both projects, that is, dividing the cash flow of project B 10 times.[10]
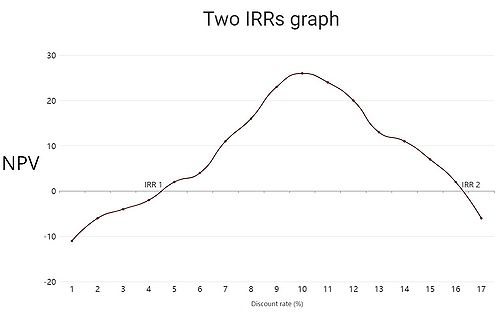
Multiple IRRs
Generally speaking, projects start with a negative cash flow, which then turns into a series of positive cash flows. For example, in the case of real estate investments, the cash flow starts negative due to the purchase of the property and over the years it becomes positive due to the income from rentals or eventual sales. This typical situation generates a change in the "direction" of the cash flows, it generates a unique IRR. But in many other projects, this change of "direction" occurs repeatedly due to negative and positive cash flows being produced alternately, this effect generates what is commonly known as multiple IRRs. To continue with the example of real estate investments, this could be the situation where it is invested in some improvements to the property which generates negative cash flows.[2]
The use of the IRR is popularly accepted for single IRR projects, because this rate clearly defines the return on an investment, being a measure of project efficiency. However, these assumptions must be carefully considered in projects with multiple IRRs.
The graph on the right represents a project with two IRRs, the first (IRR1) of 4.7% and the second (IRR2) of 16.4%. The net present value (NPV) is represented on the vertical axis and the discount rate (%) on the horizontal axis.
IRR Incalculable
The impossibility of calculating the IRR is one of the most important criticisms of this indicator. One of the situations where this problem occurs is in projects where all cash flows are positive, which rarely happens in practice (it implies output, without input). Another situation where the IRR is not calculable is when all cash flows are negative. It is clear that in these two situations there is no doubt whether the project should be accepted or rejected. The third circumstance where IRR cannot be calculated is when net cash flow is zero.[10]
Reinvestment
This limitation of the IRR is based on the "investment assumption", and this is because the IRR assumes that the positive flows generated by the project are reinvested at the IRR, not at the discount rate. And the negative flows should divest the project but these are not considered to simplify the calculation. To correct inconsistencies arising from the use of implicit assumptions, simply make them explicit. For example, if it is necessary to know what the internal rate of return of the reinvested cash flow is at the discount rate, do the calculation twice. The second time, enter the actual cash flow generated by reinvested funds for all projects. If funds are available to pay off the debt, the avoided interest is included in the project's positive cash flow. Of course, it is essential to treat all projects equally in this regard if meaningful comparisons are to be made. While this technique would have been cumbersome when writing some of the most popular textbooks decades ago, modern electronic tools make it easy. However, this adjustment is usually unnecessary. In general, reinvesting at the IRR is a reasonable assumption. This is done in the matter of bond yields, which is ultimately an investment decision.[10]
ANNOTATED BIBLIOGRAPHY
Hartman, Joseph C., and Ingrid C. Schafrick. THE RELEVANT INTERNAL RATE OF RETURN. 2004 [1]
This article clearly defines the IRR theoretically and mathematically, and also presents a method to determine the acceptability of projects with multiple IRRs. During method development, the authors explain the limitations of having multiple IRRs in a project.
References
- ↑ 1.0 1.1 1.2 Hartman, Joseph C., and Ingrid C. Schafrick. «THE RELEVANT INTERNAL RATE OF RETURN». The Engineering Economist, Vol. 49, no. 2, January 2004, p. 139-58. DOI.org (Crossref), External link.
- ↑ 2.0 2.1 2.2 2.3 2.4 Patrick, Michael, and Nick French. «The internal rate of return (IRR): projections, benchmarks and pitfalls». Journal of Property Investment & Finance, vol. 34, no. 6, January 2016, p. 664-69. Emerald Insight, External link.
- ↑ Tang, S.L., and H. John Tang. «TECHNICAL NOTE: THE VARIABLE FINANCIAL INDICATOR IRR AND THE CONSTANT ECONOMIC INDICATOR NPV». The Engineering Economist, Vol. 48, no. 1, January 2003, p. 69-78. DOI.org (Crossref), External link.
- ↑ Hazen, Gordon, and Carlo Alberto Magni. «Average Internal Rate of Return for Risky Projects». The Engineering Economist, Vol. 66, no. 2, April 2021, p. 90-120. DOI.org (Crossref), External link.
- ↑ 5.0 5.1 Barfod, M. B., & Leleur, S. (Eds.) (2014). Multi-criteria decision analysis for use in transport decision making. (2 ed.) DTU Transport. External link
- ↑ Le Maux, Benoît; Josselin, Jean-Michel. Cost Benefit Analysis, Statistical Tools for Program Evaluation — 2017, pp. 291-324 External link.
- ↑ Brown, Gerald R., and George A. Matysiak. Real Estate Investment: A Capital Market Approach. Financial Times Prentice Hall, 2000.
- ↑ 8.0 8.1 Kalhoefer, Christian. «Ranking of mutually exclusive investment projects – how cash flow differences can solve the ranking problem». Investment Management and Financial Innovations, Volume 7, Issue 2, 2010
- ↑ Shapiro, A.C. «Capital Budgeting and Investment Analysis, Upper Saddle River, 2005
- ↑ 10.0 10.1 10.2 Ray, Martin. TRIPOD: Internal Rate of Return Revisited External link. Retreived on 19 February 2022